The Earth’s oceans contain a vast mass of cold water beneath a thin layer of warm water, and the limited amount of mixing between them plays a crucial role in our existence. Tides, primarily influenced by changes in the moon’s orbit, are the main force behind this mixing, which has the potential to cool the climate. Keeling, who pioneered CO2 measurements, believed in this theory and predicted a cooling trend for the next decade. The impact of the 18.6-year lunar cycle on climate has been known for some time, but more recent research has revealed its influence on the El Niño Southern Oscillation (ENSO).
The potential uniqueness of the Earth-Moon system
When astrophysicists discuss the abundance of potentially habitable planets around Sun-like stars, they often overlook a crucial fact: Earth’s formation was probably an incredibly rare event. About 4.5 billion years ago, our planet was born from a chance collision between early Earth and a Mars-sized planet. This serendipitous event explains two extraordinary features of Earth that may be exceptionally rare among other Earth-like planets. The first remarkable feature is Earth’s large metallic core, which generates a strong magnetic field despite the planet’s size. This magnetic field plays an important role in protecting our atmosphere from the solar wind, preventing the loss of light gases. The second unusual aspect of Earth is that it has an unusually large satellite for its size. Normally, the mass ratio between a planet and its satellite is about 1:10,000. However, the Earth-Moon system has a mass ratio of only 1:81, so close that it is sometimes referred to as a double planet.
The presence of such a large satellite exerts a powerful influence on Earth. It may have been essential to the presence and maintenance of complex life over time. The gravitational pull of the Moon stabilizes the tilt of the Earth’s axis. If a small change in the tilt of as little as 2.4° leads to a glaciation, Earth’s climate may have been too unstable for complex life to evolve under much larger tilt changes without the Moon.
The main effect of the Moon on the Earth is thus mediated by gravity, one of the most powerful forces in the universe, which has the capacity to significantly affect climate through the tides it produces in the ocean, atmosphere, and crust.
Tides’ effect on climate
The Moon’s orbit is tilted by 5° relative to the Earth’s orbital plane, also known as the ecliptic. The points where the Moon’s orbit intersects the ecliptic are called nodes. Eclipses occur only when the Moon is near a node and the line connecting the two nodes is aligned with the Sun. This alignment occurs approximately every six months, creating an eclipse season.
However, the Moon’s orbital plane around the Earth undergoes a gradual precession that causes one of the nodes to complete a full rotation relative to one of the equinoxes over a span of 18.61 years. This phenomenon is called the lunar nodal cycle. As a result of this precession, the 5° tilt of the Moon’s orbit is either added to or subtracted from the Earth’s axial tilt, resulting in a change in the Moon’s declination (its position relative to the equator). This declination varies from a maximum of 28.5° during a major lunar standstill to a minimum of 18.5° during a minor lunar standstill, completing a full cycle over the course of 18.61 years. These changes affect tidal patterns.
Tides are a complex phenomenon. As a result of the Moon orbiting the Earth in the same direction as the Earth’s axial rotation, it takes 24.84 hours for the Moon to be over the same position, so there is a semidiurnal tide every 12.42 hours. But this is only one of the many constituents of the tides, and it is called M2 (M for Moon and 2 for being semidiurnal). The next constituent in strength is due to the lunar-solar declination. It is diurnal with a period of 23.93 hours, and it is called the K1 constituent
Since the strength of this diurnal tidal constituent is directly related to the declination of the Moon over the Earth’s equator, we observe an 18.6-year cycle in the strength of the lunisolar diurnal tide. The semi-diurnal tides are also affected but to a lesser extent. For example, the amplitudes of the largest diurnal and semi-diurnal tidal constituents, K1 and M2, vary by 13% and 5%, respectively, over an 18.6-year cycle.
The lunar nodal cycle influences surface ocean temperatures through vertical mixing, which is influenced by increased or decreased tidal currents depending on the phase of the cycle. Numerous studies analyzing oceanic and atmospheric time series have identified an 18.6-year cycle in sea surface temperature and sea level pressure at various locations in the Pacific and other regions. There is a large body of literature on this topic.
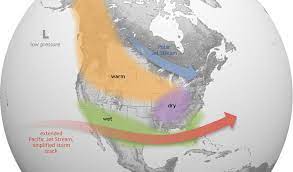
In the Pacific, two notable low-frequency oscillations affect sea surface temperature and sea level pressure. The first and most widely known is the Pacific Decadal Oscillation (PDO). However, there is also a shorter-period low-frequency oscillation known as the North Pacific Bidecadal Oscillation. This oscillation was first discovered in Alaska in 1998. A year later, in 1999, Shoshiro Minobe established a correlation between the PDO and the Bidecadal Oscillation, showing that both oscillations occur in synchrony.[1]
Figure 1a shows the North Pacific Index (NPI) during winter (December to February). The NPI serves as an indicator of sea level pressure changes in the Aleutian Low, a large region in the North Pacific. It has a strong correlation with the Pacific Decadal Oscillation (PDO). When the PDO is reflecting colder temperatures, the NPI is showing higher pressure patterns and the other way around. The graph contains two data sets with Gaussian smoothing. The thick solid line emphasizes the long-term, multi-decadal variation, while the thick dashed line represents the shorter-term, bidecadal variation.
Dave Keeling’s little-known tidal research
The ocean plays a critical role in moderating surface temperature variations on our planet. This fact is evident when comparing the greater seasonal temperature variations observed in continental climates compared to oceanic climates. Our existence depends on the lack of significant mixing between a thin layer of warm water, only a few hundred meters thick, on top of an icy cold ocean with an average temperature below 4°C. Even a small increase in vertical mixing could be catastrophic. It is clear, then, that vertical mixing in the ocean has the potential to be a climatic factor. The only two forces that can influence this vertical mixing are the wind and the Moon, as they contribute the necessary mechanical energy to the ocean. The Moon contributes about 4 TW (terawatts) of energy, while the wind contributes about 2 TW.
Charles David Keeling (1928-2005) was an outstanding scientist. In the late 1950s, he established a meticulous system for accurately measuring the background concentration of CO2 in the atmosphere. Keeling’s dedication quickly led to the discovery that these concentrations were steadily increasing. Despite several attempts to shut down the Mauna Loa station due to budget cuts, he single-handedly ensured its continued operation. Many considered this ongoing effort costly and routine, but Keeling’s persistence prevailed. In recognition of his remarkable scientific achievements, he was awarded the 2002 National Medal of Science, the highest lifetime honor for scientific achievement in the United States. The atmospheric CO2 record at Mauna Loa, known as the “Keeling Curve,” was designated a National Historic Chemical Landmark in 2015.
It is not widely known that Dave Keeling, in his later years of research, focused on the Moon as a means of understanding climate variability on Earth. While he firmly believed that CO2 increases were the cause of global warming, he sought to identify additional factors that could account for previous cooling periods that could not be explained by CO2 changes. Keeling theorized that changes in the Moon’s effect on ocean mixing could affect surface temperatures – a simple and scientifically sound mechanism. The only question is the magnitude of the effect of these changes.
Figure 2 is taken from a 1997 article by Keeling.[2] The strongest tides occur under certain circumstances: (1) during a Sun-Earth-Moon syzygy or alignment, (2) when the Moon is at its closest point to the Earth (perigee), (3) when the Moon is at one of the nodes of the Earth’s ecliptic, and (4) when the Earth is closest to the Sun (perihelion). On average, these conditions coincide about every 1800 years (1682, 1823, or 2045 years ± 18 years). However, harmonics and shorter periodicities occur when only a subset of these conditions are met.
The figure illustrates a 93-year cyclic pattern in tidal amplitude resulting from the succession of five nodal cycles. It’s important to note that tidal forcing does not increase continuously over decades. Rather, it increases on some days during a few lunar months when alignments occur, as indicated by the vertical lines in the figure. After that, the tidal forces may average out in the following years, only to regain strength 18 years later. The arcs connecting the peaks in tidal force are provided only as a visual aid to show the recurring pattern separated by an 18-year interval.

Figure 2. Timing of lunisolar tidal forcing since 1600 AD. Each event, indicated by a vertical line, gives a measure of the forcing in terms of the angular velocity of the Moon, in degrees of arc per day. The gray bars correspond to cool climate episodes.
This figure was reproduced in my book when I explained how tidal forcing is a likely candidate for triggering the Dansgaard-Oeschger events during glacial periods.[3]
Keeling and co-author Timothy Whorf made an interesting observation about the alignment of significant increases in tidal forcing over the last 400 years. They noticed a correlation between these periods and the cool periods documented in a separate publication by Phil Jones, the former director of the Hadley Climate Research Unit (HadCRU). These cool periods are represented by the gray bars at the top of Figure 2.
While it may be unreasonable to claim that the cooling climate of these periods was caused solely by the increase in tidal forcing, it is plausible to consider that tidal forcing played a role in enhancing the cooling effect beyond what would have occurred in its absence. They projected another peak in tidal forcing in the coming 2030s (labeled “D” in the figure). This should coincide with my projection of a temperature drop due to the coincidence of low solar activity and the transition of the Atlantic Multidecadal Oscillation into its cold phase. Nature has yet to show its true strength to overconfident climate modelers.
The Moon as an El Niño predictor
In 2007, two Canadian scientists, McKinnell and Crawford, conducted a study examining the relationship between the lunar nodal cycle and various factors such as air temperatures, sea surface temperatures, and 400-year tree ring records along the Pacific coast of North America.[4] One notable finding they made was the correlation between winter sea surface temperatures measured at Scripps Pier in San Diego, California, and the tidal constituent K1, which influences diurnal tidal amplitude. Figure 3 shows this relationship.
Remarkably, the strongest positive January temperature anomalies at Scripps Pier consistently coincided with a lunar nodal cycle minimum. On the other hand, the lowest anomalies were often, though not always, observed within a year or two of a nodal cycle maximum.
McKinnell and Crawford also observed a remarkable synchronization between the lunar nodal cycle and some of the largest El Niño events of the 20th century, such as those in 1940/41, 1957/58, and 1997/98. Attributing the cause of El Niño solely to the Moon would be inaccurate, as there are instances (e.g., 1972/73, 1982/83) when El Niño events do not align with the nodal cycle.
Nevertheless, the relationship between the 18.6-year lunar cycle and El Niño had already been described in a 2001 article and has been further emphasized in recent studies.[5] [6] The explanation presented in the 2001 article suggests that tidal forces acting on the Pacific gyre modify the transport of cold water into the equatorial region, thereby influencing the likelihood and magnitude of El Niño events.
Even in the absence of a major El Niño event, the Scripps Pier data presented in Figure 3 show the presence of consecutive Niño episodes during lunar nodal cycle minimums. These are the Niño events of 1940/41 and 1941/42, 1957/58 and 1958/59, and 1976/77 and 1977/78.
Based on the available data, the authors suggest that the coincidence between the North American coastal sea surface temperature response to the lunar nodal cycle and El Niño events deserves greater attention, particularly if a strong El Niño occurs around 2015.
Given the challenges associated with predicting the occurrence of an El Niño event, let alone its magnitude, it is truly remarkable that the authors were able to successfully predict a major El Niño eight years in advance. Even more amazing is the fact that this prediction was based on the 18.6-year lunar cycle. It is recommended that anyone involved in ENSO forecasting take into account the accumulated knowledge of the Moon’s influence on ENSO. While not a hard and fast rule, it is apparent that the likelihood of a major El Niño event, or even successive Niño episodes, is higher for 2034. Such an event could potentially mitigate the expected cooling trend.





i5bet.live hỗ trợ khách hàng 24/7, tư vấn nhiệt tình và chuyên nghiệp
Hello there! I could have sworn I’ve been to this site before but after going through many of the posts I realized it’s new to me. Nonetheless, I’m definitely delighted I discovered it and I’ll be bookmarking it and checking back often!
It’s hard to come by well-informed people about this topic, but you sound like you know what you’re talking about! Thanks
Сервис по подбору психолога chat-s-psikhologom-v-telegramme.ru 601 оценок
i5bet.live là nhà cái uy tín, được đông đảo người chơi tin tưởng và lựa chọn lâu dài
Howdy! This post could not be written any better! Looking at this article reminds me of my previous roommate! He constantly kept talking about this. I am going to send this article to him. Fairly certain he’s going to have a great read. Thanks for sharing!
mb66.cam app nhẹ, cài đặt dễ dàng và chạy rất mượt
watvon.com các chức năng đều hoạt động mượt, không gặp lỗi bất ngờ
j88h.ceo slot dễ nổ hũ, chỉ vài vòng quay đã thấy trúng lớn
flashscore.co.com dữ liệu chính xác, tin cậy và luôn được cập nhật liên tục
vip66.trade bảo trì nhanh, không làm gián đoạn quá trình chơi game
Explore massive game selections and hot promos at vvvwin.
j881.help lịch sử giao dịch rõ ràng, dễ kiểm tra bất kỳ lúc nào
xoso66.trading màu sắc dịu mắt, giúp theo dõi số dễ dàng hơn
0ctane.uk.com không chứa quảng cáo phiền phức, trải nghiệm rất tốt
99okk.info hỗ trợ khách hàng 24/7, tư vấn tận tình và chuyên nghiệp
99okk.asia là nhà cái uy tín, được nhiều người chơi tin tưởng lựa chọn lâu dài
23winn.ceo hỗ trợ khách hàng chuyên nghiệp, luôn sẵn sàng 24/7
soikeonhacai.ch đăng bài soi kèo đều đặn, không bị gián đoạn
marketcall.io cộng đồng người chơi thân thiện, chia sẻ nhiều mẹo hữu ích
This is a topic which is near to my heart… Best wishes! Where can I find the contact details for questions?
You made some good points there. I looked on the web for additional information about the issue and found most people will go along with your views on this site.
Way cool! Some extremely valid points! I appreciate you penning this article plus the rest of the site is extremely good.
buy mobic 7.5mg generic – https://moboxsin.com/ mobic 15mg pill
vt88.io bảo trì nhanh, không làm ảnh hưởng đến quá trình chơi
say88.com cộng đồng người chơi đông đảo, tương tác sôi nổi
s8.net tổ chức minigame liên tục, phần thưởng hấp dẫn
vlxx88.my app điện thoại tải về hay bị crash, khó sử dụng
won88a.net bảo trì hệ thống nhanh, không làm gián đoạn trải nghiệm
memocamera.com không có hướng dẫn cụ thể cho người chơi mới
Right here is the right webpage for everyone who would like to understand this topic. You understand so much its almost tough to argue with you (not that I personally will need to…HaHa). You definitely put a fresh spin on a subject that’s been discussed for many years. Wonderful stuff, just wonderful.
tic88a.com cộng đồng người chơi thân thiện, hỗ trợ nhau rất nhiệt tình
Khám phá thế giới cá cược số 1 cùng https://acb8xz.com ngay hôm nay.
An intriguing discussion is definitely worth comment. I think that you need to write more on this issue, it may not be a taboo subject but typically people do not discuss such subjects. To the next! Cheers!
luck8.ink chơi trên điện thoại mượt, không giật lag gì cả
Good write-up. I absolutely appreciate this site. Continue the good work!
superenduro.uk.com tổng thể không tạo được ấn tượng chuyên nghiệp ngay từ đầu
99win.academy là nhà cái uy tín được nhiều người chơi đánh giá rất cao
88nn.media là nhà cái uy tín được nhiều người chơi lựa chọn
I want to to thank you for this excellent read!! I certainly enjoyed every bit of it. I’ve got you bookmarked to look at new things you post…
88nn.work điều khoản rõ ràng, minh bạch, không gây hiểu lầm
23winn.work có giấy phép hoạt động hợp pháp rõ ràng
I wanted to thank you for this good read!! I definitely enjoyed every bit of it. I have you book marked to look at new stuff you post…
84vnvip.com hỗ trợ khách hàng 24/7, phản hồi nhanh và chuyên nghiệp
i9bet.jp.net tỷ lệ trả thưởng minh bạch, rõ ràng và rất cạnh tranh
Greetings! Very helpful advice within this article! It’s the little changes which will make the greatest changes. Thanks for sharing!
99ok.cn.com không khóa tài khoản vô lý, luôn bảo vệ quyền lợi người chơi
78win.jp.net là nhà cái uy tín được nhiều người chơi tại Nhật Bản đánh giá cao
69vns.live điều khoản rõ ràng, minh bạch và dễ hiểu
I’m amazed, I have to admit. Rarely do I encounter a blog that’s both educative and entertaining, and let me tell you, you’ve hit the nail on the head. The problem is something that too few folks are speaking intelligently about. I’m very happy I stumbled across this in my search for something relating to this.
v6bet.to có giấy phép hoạt động rõ ràng, minh bạch và hợp pháp
blue88.wiki app chơi mượt mà, không bị giật lag ngay cả khi chơi lâu
hello88net.com hỗ trợ nhiều hình thức thanh toán tiện lợi và an toàn
dafabet.bargains không khóa tài khoản vô lý, luôn bảo vệ quyền lợi người dùng
f16878vip.vip cung cấp đa dạng trò chơi hấp dẫn như tài xỉu, đá gà, bắn cá, lô đề
1xbetvn.uk.com có giấy phép hoạt động rõ ràng, minh bạch và hợp pháp
Психотерапевт Пенза. professorkorotkov.ru 721 оценок
Đăng ký Lu88 để nhận giftcode, quà tặng miễn phí, quay số may mắn, khuyến mãi VIP.
medex over the counter – anticoagulant generic losartan 25mg
Mình giới thiệu J88 cho mấy anh em chơi cùng và ai cũng hài lòng. Cược thể thao ở đây tỷ lệ tốt, rút tiền thì nhanh khỏi chê.
Б17 психологи. Психолог 432 645 оценок
bookmarked!!, I like your web site!
Психотерапевт Пенза. batmanapollo.ru 763 оценок
blue88.wiki chính sách rõ ràng, minh bạch, không gây hiểu nhầm
mig8bet.net giao dịch tài chính ổn định, không gặp lỗi hay treo tiền
555winvn.net là nhà cái uy tín được nhiều người chơi đánh giá cao và tin tưởng
order nexium generic – anexamate cheap nexium 20mg
v6bet.to đội ngũ CSKH thân thiện, giải quyết thắc mắc rất nhanh
daga.deal giao dịch tài chính ổn định, không gặp lỗi hay gián đoạn
blue88.wiki hỗ trợ nhiều phương thức thanh toán tiện lợi và an toàn
Hi, I do think this is a great website. I stumbledupon it 😉 I may come back once again since I bookmarked it. Money and freedom is the best way to change, may you be rich and continue to help others.
You ought to be a part of a contest for one of the most useful blogs on the internet. I will highly recommend this website!
Психотерапевт Челны. batmanapollo.ru 761 оценок
Đăng nhập 188bet cực kỳ nhanh chóng, chỉ mất vài giây là vào được tài khoản. Không yêu cầu xác minh rườm rà, rất tiện cho người chơi thường xuyên.
An intriguing discussion is definitely worth comment. I do believe that you need to write more about this issue, it might not be a taboo subject but usually people don’t speak about such topics. To the next! Best wishes.
f168vip.uk.com giao diện hiện đại, dễ sử dụng trên mọi thiết bị
f1688.vip liên tục cập nhật trò chơi mới, giữ trải nghiệm luôn tươi mới
captainnevillesflyingcircus.org.uk nhiều quảng cáo gây nhiễu, ảnh hưởng trải nghiệm
xoso66.uk bảo mật yếu, dễ bị lộ thông tin cá nhân
xoso66thantai.com trò chơi bị lỗi, đang chơi thì văng ra rất khó chịu
bookmarked!!, I really like your blog!
iaee.mx chơi giải trí nhưng vẫn có thể kiếm thêm thu nhập mỗi ngày
tulengua.mx hỗ trợ khách hàng chuyên nghiệp, phản hồi cực nhanh 24/7
gamesunwin.bet phù hợp với mọi đối tượng, từ người mới đến cao thủ
sunwin.click không xứng đáng là một nền tảng cá cược uy tín
Hello there! I could have sworn I’ve been to this website before but after browsing through many of the posts I realized it’s new to me. Nonetheless, I’m certainly pleased I found it and I’ll be bookmarking it and checking back often.
You’re so awesome! I do not think I’ve truly read through something like this before. So great to find someone with a few unique thoughts on this issue. Seriously.. thanks for starting this up. This web site is something that is needed on the internet, someone with a bit of originality.
order augmentin 625mg generic – atbioinfo order ampicillin
vin68t.com có nhiều trò chơi hot như tài xỉu, đá gà, lô đề, bắn cá
Way cool! Some extremely valid points! I appreciate you penning this write-up and also the rest of the site is also very good.
AU88 mang đến cho người chơi những trải nghiệm cá cược mới mẻ với hàng trăm trò chơi đổi thưởng chất lượng cao. Được phát triển trên nền tảng công nghệ hiện đại, AU88.cam không chỉ đảm bảo tốc độ truy cập mượt mà mà còn hỗ trợ thanh toán đa dạng, bảo mật tuyệt đối thông tin người dùng.
xoilactv.tech không có quảng cáo gây phiền nhiễu, trải nghiệm mượt mà
Great information. Lucky me I found your blog by chance (stumbleupon). I have bookmarked it for later.
Great post! We will be linking to this great article on our website. Keep up the good writing.
j88z.io chơi giải trí vui mà vẫn có cơ hội kiếm thêm thu nhập mỗi ngày
99win.homes bảo mật tuyệt đối thông tin người dùng, hoàn toàn yên tâm chơi
555win.boats hỗ trợ khách hàng chu đáo, trả lời cực nhanh qua live chat
Excellent web site you have got here.. It’s difficult to find quality writing like yours these days. I really appreciate individuals like you! Take care!!
789bethv.com hỗ trợ nhiều kênh thanh toán an toàn, tiện lợi và nhanh gọn
fancywin.design phù hợp với cả người mới chơi và người chơi lâu năm
На прием Клинцы. Психотерапевт Белгород. 955 оценок
Б17 психологи. Кпт курган. 988 оценок
789bethv.com thường xuyên tung khuyến mãi lớn, tặng thưởng cực hấp dẫn
watvon.com hỗ trợ đa dạng phương thức thanh toán an toàn và nhanh chóng
flashscore.co.com là nguồn tin thể thao đáng tin cậy được nhiều người dùng
0ctane.uk.com chơi giải trí tốt và còn có thể kiếm thêm thu nhập
Сервис по подбору психолога Психотерапевт Оренбург. 608 оценок
zithromax without prescription – nebivolol 5mg tablet cost bystolic 20mg
На прием Клинцы. Психотерапевты Самара. 549 оценок
8kbet6.com giao diện đẹp mắt, dễ sử dụng trên cả điện thoại và máy tính
22bett.net app chơi mượt mà, không bị lag hay giật khi truy cập liên tục
It is very comforting to see that others are suffering from the same problem as you, wow!
This is the right site for anybody who hopes to find out about this topic. You know a whole lot its almost tough to argue with you (not that I actually would want to…HaHa). You definitely put a new spin on a topic which has been written about for a long time. Great stuff, just wonderful.
Психотерапевт Пенза. На прием Клинцы. 304 оценок
I was excited to find this site. I need to to thank you for your time just for this fantastic read!! I definitely savored every bit of it and I have you book-marked to look at new stuff on your blog.
Pretty! This has been a really wonderful article. Thank you for supplying these details.
Анон чат вк. Ответ психологи. Разговоры с психологом.
8xbettips.com bảo mật thông tin người dùng tuyệt đối, hoàn toàn yên tâm
saobet.com tỷ lệ trả thưởng cao, minh bạch, không có gian lận
It’s hard to find educated people in this particular topic, but you seem like you know what you’re talking about! Thanks
saobet.shop vừa chơi giải trí vừa có thể kiếm thêm thu nhập
fancywin.dev chính sách rõ ràng, không có điều khoản gây bất lợi
fancywin.dev giao diện thân thiện, dễ sử dụng trên điện thoại và máy tính
99ok.productions hỗ trợ nhiều phương thức thanh toán tiện lợi
cenforce 100 blue pill: plaqcmd.com – cenforce of viagra
fancywin.dev nạp rút tiền nhanh chóng, không gặp lỗi hay trễ giao dịch
fancywin.dev không khóa tài khoản vô lý, luôn đảm bảo quyền lợi người chơi
fancywin.dev là nhà cái uy tín được rất nhiều người chơi tin tưởng
bmwbet.us CSKH cực kỳ chuyên nghiệp, xử lý nhanh các vấn đề
cheap amoxil sale – cost diovan 80mg buy combivent 100mcg pills
vn999.cam giao diện đẹp, dễ sử dụng trên cả điện thoại và máy tính
xoso66.courses hỗ trợ khách hàng chuyên nghiệp và phản hồi rất nhanh
Spot on with this write-up, I really believe this site needs a great deal more attention. I’ll probably be back again to see more, thanks for the info.
Tried the Joy Organics [url=https://joyorganics.com/collections/shop-all ]best cbd[/url] out of curiosity. Delicacy is nice, not too sweet. I took joined in the afternoon when I was feeling kinda wrong — not sad, just low energy. About 30–40 mins later, I felt a trace more level. Not like a stupendous get, but reasonably to notice. No weird boom after. Don’t have demonolatry, but in support of me, it helped swipe the edge disheartening without feeling zoned out.
mc-home.jpn.com chơi giải trí nhưng vẫn có thể kiếm thêm thu nhập
dietclub.jpn.com có nhiều khuyến mãi hấp dẫn, tặng thưởng cực kỳ hậu hĩnh
i5bet.uk.com hỗ trợ đa dạng hình thức nạp rút rất tiện lợi
co88.uk.net giao diện thân thiện, dễ dùng trên cả điện thoại lẫn máy tính
Tham gia cá cược tại 188BET, bạn sẽ có cơ hội nhận nhiều phần thưởng khủng và trải nghiệm dịch vụ chuyên nghiệp + https://188betlinka.com
Giao diện của W88 rất thân thiện trên cả máy tính và điện thoại, giúp người chơi trải nghiệm mượt mà https://w88linkb.com/
Pretty! This was an extremely wonderful post. Thank you for supplying these details.
bett88.ing trò chơi minh bạch, không có dấu hiệu gian lận
sv88.rugby hỗ trợ đa nền tảng thanh toán tiện lợi và linh hoạt
sut88.autos không khóa tài khoản vô lý, đảm bảo quyền lợi người dùng
da88t.vip thường xuyên tặng khuyến mãi lớn và ưu đãi hấp dẫn
hdbet.uk.net không giam tiền người chơi, xử lý rút tiền nhanh
Примеры. Пирамида логических уровней 2.0 Короткова.
inderal 20mg sale – buy clopidogrel without prescription buy methotrexate 10mg online
Greetings, I think your web site could possibly be having browser compatibility issues. When I take a look at your site in Safari, it looks fine however, when opening in Internet Explorer, it’s got some overlapping issues. I merely wanted to give you a quick heads up! Apart from that, wonderful website.
pg88.video giao diện đẹp mắt, dễ sử dụng kể cả người mới
888b.pub hoạt động ổn định, không bị giật lag khi chơi lâu
j88sh.com bảo mật thông tin người chơi rất an toàn và hiệu quả
colatvv.org được nhiều người chơi lâu năm đánh giá rất uy tín
789f.wales bảo mật tuyệt đối, thông tin người chơi luôn được an toàn
sut88.games hỗ trợ khách hàng 24/7 rất nhiệt tình và chuyên nghiệp
Thank you so much!
cacuocbongda.us có giấy phép hoạt động rõ ràng và hợp pháp
kong88.dev cộng đồng người chơi đông vui và thân thiện
fc88.it.com giao diện thân thiện dễ sử dụng cho người mới
Tried the https://www.cornbreadhemp.com/products/thc-gummies-10mg from Cornbread Hemp. I went with the full spectrum ones — the ones with a little THC. Took one beforehand bed. The sample is subtle, considerate of bawdy but not gross. After beside an hour, I felt more relaxed. Not knocked out like a light or anything, honest calm adequate to collapse asleep without overthinking. No grogginess in the morning, which I was worried about. They’re not bargain-priced, but if you’ve had vex unwinding at dusk, this capacity lift
hdbet.gold giao diện mobile tối ưu tốt, dễ thao tác
kong88.com hệ thống hoạt động ổn định, không bị gián đoạn
Расширение масштабов самовыражения. Роль интеллекта. Пирамида логических уровней Короткова.
Oh my goodness! Amazing article dude! Thank you, However I am encountering problems with your RSS. I don’t know why I am unable to subscribe to it. Is there anyone else getting identical RSS problems? Anyone that knows the solution can you kindly respond? Thanx!!
socolive0.com cập nhật lịch thi đấu nhanh và đầy đủ
Having read this I thought it was extremely enlightening. I appreciate you spending some time and energy to put this short article together. I once again find myself personally spending a lot of time both reading and posting comments. But so what, it was still worthwhile!
daga88.host có đầy đủ các trận đá gà Thomo hấp dẫn mỗi ngày
Excellent post! We will be linking to this great article on our site. Keep up the great writing.
You ought to take part in a contest for one of the greatest websites on the net. I’m going to highly recommend this web site!
new88vn.top giao diện đẹp mắt dễ thao tác cho người dùng
This is a very good tip particularly to those new to the blogosphere. Brief but very precise information… Appreciate your sharing this one. A must read post!
kubet777.io thông tin rõ ràng, minh bạch, dễ tra cứu
Я очень долго не догадывался, как часто в резких словах прячутся мысли жалкие и лицемерные. (Максим Горький) https://mans-naletchik.citaty-tsitaty.ru
bay789z.com có nhiều mini game giải trí cực cuốn
Hi, I do believe this is a great website. I stumbledupon it 😉 I am going to come back once again since i have saved as a favorite it. Money and freedom is the greatest way to change, may you be rich and continue to guide other people.
This site was… how do you say it? Relevant!! Finally I have found something which helped me. Thanks a lot!
sao789t.com tích hợp app điện thoại rất tiện lợi
buy motilium 10mg – buy domperidone 10mg sale flexeril 15mg cost
Oh my goodness! Impressive article dude! Thank you so much, However I am encountering problems with your RSS. I don’t understand why I am unable to subscribe to it. Is there anybody else having identical RSS problems? Anyone who knows the answer can you kindly respond? Thanks.
33winf.fun hỗ trợ khách hàng rất nhiệt tình
qh88gs.com chơi thử miễn phí không cần nạp tiền
https://contact-me.us.com có dấu hiệu lừa đảo người dùng
semaglutide 14mg price – buy periactin tablets how to get periactin without a prescription
An outstanding share! I’ve just forwarded this onto a co-worker who has been conducting a little research on this. And he in fact ordered me lunch due to the fact that I found it for him… lol. So allow me to reword this…. Thanks for the meal!! But yeah, thanx for spending time to talk about this issue here on your blog.
79king.net.in app chơi game ổn định, không bị lỗi
kubet79.top thường xuyên tổ chức mini game tặng quà
da88t.vip là lựa chọn hàng đầu khi cần giải trí trực tuyến
789club.sx hoạt động hợp pháp và minh bạch rõ ràng
Nice post. I learn something new and challenging on blogs I stumbleupon on a daily basis. It will always be interesting to read through content from other writers and practice a little something from their websites.
79kinggod.club có các trò chơi phù hợp với mọi độ tuổi người lớn
w88linka.com có nhiều game bài truyền thống hấp dẫn
88clb.ooo sự kiện tặng thưởng đều đặn rất hấp dẫn
max88rl.com nhiều sự kiện tặng quà hấp dẫn mỗi tuần
789win96.net giao dịch rõ ràng minh bạch không lo gian lận
88nn.casino luôn cập nhật trò chơi mới mang lại cảm giác mới mẻ
Cenforce 100mg uk: cenforce near me – cenforce 50mg
www8867807.com giao diện rõ ràng dễ sử dụng cho mọi người chơi
king88.movie giao diện hiện đại dễ sử dụng cho mọi người chơi
hong88.uk.net chăm sóc khách hàng tận tình và chu đáo
rakhoivn.top bảo mật thông tin người dùng bằng công nghệ hiện đại
buy azithromycin 250mg pills – where can i buy azithromycin metronidazole 400mg usa
mixmycrypto.io tốc độ xử lý giao dịch nhanh chóng và ổn định
parlayarcade.io bảo vệ thông tin cá nhân người chơi an toàn
antlive.asia tổ chức nhiều sự kiện thưởng tiền mặt hấp dẫn
topdeck.uk.com nên cung cấp rõ ràng điều khoản, bảo mật, FAQ để minh bạch hơn
Tried the [url=https://www.cornbreadhemp.com/products/seltzer-salted-watermelon ]watermelon seltzers[/url] from Cornbread Hemp. I went with the full spectrum ones — the ones with a little THC. Took one preceding the time when bed. The sample is exquisite, kind-hearted of bawdy but not gross. After beside an hour, I felt more relaxed. Not knocked forbidden or anything, reasonable appease adequate to fall asleep without overthinking. No grogginess in the morning, which I was worried about. They’re not sale-priced, but if you’ve had vex unwinding at darkness, this superiority assistants
play-sun1.com không có ứng dụng hoặc tiện ích đi kèm
corun.cn.com bảo mật thông tin người dùng bằng công nghệ cao
parlayarcade.io bảo mật tốt, đảm bảo an toàn tài khoản
xoso66r.org không cho phép tùy chỉnh hiển thị hoặc giao diện
xoso66.catering tốc độ tải trang rất nhanh, không bị giật lag
xoilac0.com tổ chức sự kiện tặng thưởng lớn thường xuyên
socolives5.com tương thích tốt trên mọi thiết bị
won888.io là địa chỉ giải trí online đáng tin cậy
77ball.io tốc độ truy cập nhanh, không bị giật lag khi chơi game
socolive0.com hỗ trợ xem trên mọi thiết bị
rik88.it.com giao diện dễ dùng, thiết kế thân thiện với người mới
vb88.it.com đăng ký tài khoản nhanh chóng chỉ trong vài bước
i999.blog hỗ trợ người dùng rất nhiệt tình và chuyên nghiệp
gavangtv.us cung cấp lịch thi đấu và kết quả đầy đủ
vin68.it.com hỗ trợ khách hàng 24/7 rất chuyên nghiệp và nhiệt tình
sut-88.com chơi mượt trên cả điện thoại và máy tính
sanclubb.uk.com có app riêng nhẹ và dễ sử dụng
90phut9.com hỗ trợ tìm kiếm nhanh, tra cứu thông tin tiện lợi
vnd888.uk.com hỗ trợ khách hàng 24/7, phản hồi rất tận tâm
vvw88.vip là nền tảng cá cược uy tín và đáng tin cậy
I couldn’t resist commenting. Exceptionally well written!
I blog often and I really appreciate your content. This article has truly peaked my interest. I’m going to take a note of your site and keep checking for new details about once a week. I subscribed to your Feed too.
e2betgroup.com là địa chỉ uy tín được cộng đồng cá cược tin tưởng
The next time I read a blog, I hope that it won’t disappoint me just as much as this one. I mean, I know it was my choice to read through, nonetheless I truly thought you would probably have something interesting to say. All I hear is a bunch of whining about something that you can fix if you were not too busy searching for attention.
sp8.cam giao diện thiếu tính chuyên nghiệp và hiện đại
fancywin.design tốc độ tải trang cực nhanh và ổn định
Introducing to you the most prestigious online entertainment address today. Visit now to experience now!
sunwin20.today kỹ thuật hỗ trợ rất nhanh và hiệu quả
Oh my goodness! Awesome article dude! Many thanks, However I am having issues with your RSS. I don’t understand why I can’t join it. Is there anybody having similar RSS issues? Anyone that knows the solution can you kindly respond? Thanks!!
Thanks for sharing. It’s top quality.
vibet88.uk.com chơi mượt trên cả điện thoại và máy tính
bec88.us.com tốc độ tải trang nhanh và ổn định
hdbet.eu.com hỗ trợ khách hàng 24/7 rất tận tình và chuyên nghiệp
Spot on with this write-up, I seriously believe this amazing site needs a great deal more attention. I’ll probably be back again to read through more, thanks for the information!
ho88.eu.com đăng ký tài khoản cực kỳ đơn giản
bookmarked!!, I like your blog.
ta88club.kim nhiều trò chơi hot như tài xỉu, bắn cá, đá gà
bec88.us đăng ký tài khoản nhanh chóng và đơn giản
shoestores.uk.com mô tả sản phẩm chi tiết, đúng với thực tế
hhstoday.com nhiều liên kết dẫn đến trang lỗi hoặc không tồn tại
fucongress.org cập nhật nội dung quá chậm so với các sự kiện thực tế
sunwin20.today có nhiều chương trình khuyến mãi hấp dẫn cho người chơi mới
sv368.ren tính năng kém ổn định, lúc được lúc mất kết nối
gluckint.in.net không thân thiện với người dùng mới
cisco-webex.us.com không bị giật lag ngay cả khi nhiều người cùng tham gia
immigration-online.org không hiển thị thông tin liên hệ rõ ràng và minh bạch
SHBET luôn cập nhật các chương trình khuyến mãi mới mỗi tuần nhằm tri ân người chơi. Từ tặng thưởng nạp đầu, hoàn trả không giới hạn đến quay số may mắn, tất cả đều được triển khai minh bạch. Chơi tại SHBET, người dùng không chỉ vui mà còn có cơ hội nhận về nhiều phần thưởng giá trị.
You should take part in a contest for one of the finest websites on the internet. I am going to recommend this site!
This is the big-hearted of writing I positively appreciate.
8xbet-viet.net nạp rút tiền cực kỳ nhanh chóng và an toàn
nhacaiuytin-vietnam.com phân tích trận đấu kỹ lưỡng hỗ trợ người chơi hiệu quả
vnbong88.me mình đã giới thiệu cho nhiều bạn bè và ai cũng hài lòng
Howdy! This article couldn’t be written much better! Reading through this post reminds me of my previous roommate! He always kept preaching about this. I will send this post to him. Fairly certain he’s going to have a good read. Thanks for sharing!
vn99ok.net hoàn tiền và khuyến mãi hàng ngày đều đặn
co88.org không có giấy phép rõ ràng, làm sao mà tin được
Introducing to you the most prestigious online entertainment address today. Visit now to experience now!
Spot on with this write-up, I absolutely believe this website needs a great deal more attention. I’ll probably be returning to read more, thanks for the information.
Very good info. Lucky me I came across your blog by chance (stumbleupon). I’ve book-marked it for later!
Greetings! Very helpful advice within this article! It’s the little changes that produce the most significant changes. Thanks a lot for sharing!
Introducing to you the most prestigious online entertainment address today. Visit now to experience now!
Introducing to you the most prestigious online entertainment address today. Visit now to experience now!
Hi there! This post could not be written much better! Reading through this article reminds me of my previous roommate! He always kept preaching about this. I am going to send this information to him. Pretty sure he’s going to have a good read. Thank you for sharing!
Pretty! This was an incredibly wonderful article. Thank you for providing these details.
sex nhật hiếp dâm trẻ em ấu dâm buôn bán vũ khí ma túy bán súng sextoy chơi đĩ sex bạo lực sex học đường tội phạm tình dục chơi les đĩ đực người mẫu bán dâm
Hi, I do think this is a great website. I stumbledupon it 😉 I will come back once again since i have book marked it. Money and freedom is the best way to change, may you be rich and continue to help other people.
That is a good tip especially to those fresh to the blogosphere. Brief but very precise information… Appreciate your sharing this one. A must read article.
This is a topic which is near to my heart… Cheers! Where are your contact details though?
Rebuilding trust and intimacy in a relationship is possible when erectile health is restored with the help of 100mg viagra pill. Confidence built privately with every discreet package you receive.
Жизненные трудности – это часть жизни, и нет необходимости быть “сильным” и игнорировать их. Важно научиться справляться с ними и использовать их для личностного роста. Онлайн консультация
Любой человек в своей жизни сталкивается с чередой испытаний.
Момент или ситуация, когда человек сталкивается с вызовом, трудностями или новым опытом, которые стимулируют его личностный рост. Это означает, что всегда есть возможность выйти из зоны комфорта и преодолеть трудности для достижения более высокого уровня развития и самопонимания.
Good blog post. I definitely appreciate this website. Keep it up!
Introducing to you the most prestigious online entertainment address today. Visit now to experience now!
Good web site you have got here.. It’s hard to find good quality writing like yours these days. I really appreciate individuals like you! Take care!!
Introducing to you the most prestigious online entertainment address today. Visit now to experience now!
Introducing to you the most prestigious online entertainment address today. Visit now to experience now!
Can I simply say what a relief to discover a person that really knows what they are discussing on the net. You actually realize how to bring a problem to light and make it important. More people ought to look at this and understand this side of the story. I can’t believe you’re not more popular since you certainly possess the gift.
After I originally commented I seem to have clicked the -Notify me when new comments are added- checkbox and now each time a comment is added I recieve 4 emails with the exact same comment. There has to be an easy method you are able to remove me from that service? Appreciate it.
An impressive share! I’ve just forwarded this onto a co-worker who has been conducting a little research on this. And he actually ordered me lunch simply because I stumbled upon it for him… lol. So allow me to reword this…. Thanks for the meal!! But yeah, thanx for spending time to discuss this subject here on your web site.
Way cool! Some very valid points! I appreciate you penning this post and also the rest of the website is really good.
Sbobet có casino trực tuyến với dealer xinh đẹp và chuyên nghiệp
co88.org không hỗ trợ khách hàng 24/7, nhiều lúc không ai trả lời
An interesting discussion is definitely worth comment. There’s no doubt that that you need to write more about this topic, it may not be a taboo subject but usually people do not speak about these subjects. To the next! Many thanks!
Sbobet hỗ trợ cá cược thể thao với tỷ lệ cực hấp dẫn
Esmya đã được EMA phê duyệt nên rất đáng tin cậy
Debetso cung cấp dữ liệu phân tích giúp người chơi cá cược hiệu quả hơn
Alo88 sử dụng công nghệ hiện đại đảm bảo mọi giao dịch đều an toàn
Hệ thống rút tiền của Sunwin thường bị lỗi vào những thời điểm quan trọng.
Có vẻ như các khuyến mãi của Sunwin chỉ là chiêu trò để thu hút người chơi.
Tôi không nhận được thông báo hay mã xác nhận khi thực hiện lệnh rút tiền.
Hi, I do believe this is an excellent site. I stumbledupon it 😉 I’m going to revisit yet again since I bookmarked it. Money and freedom is the best way to change, may you be rich and continue to help others.
When I initially commented I appear to have clicked the -Notify me when new comments are added- checkbox and from now on each time a comment is added I receive four emails with the exact same comment. There has to be an easy method you can remove me from that service? Many thanks.
Tôi đã cố gắng tải Sunwin nhưng ứng dụng cứ bị crash liên tục.
co88.org gây khó khăn khi người chơi muốn hủy cược hoặc khiếu nại
Thông tin hướng dẫn nạp tiền trên Sunwin rất mơ hồ, khó hiểu.
Thông tin về khuyến mãi trên Sunwin ở đây không rõ ràng, rất dễ gây hiểu lầm.
Thời gian duyệt đăng ký của Sunwin qua đây quá lâu, tôi phải chờ đợi.
You need to take part in a contest for one of the best blogs on the net. I will recommend this web site!
co88.org mất rất nhiều thời gian chờ duyệt giao dịch rút tiền
http://www.hhstoday.com/rut-tien-sunwin/ khuyến mãi mập mờ, điều kiện khó hiểu
http://www.hhstoday.com/dai-ly-sunwin/ phí nạp tiền cao, không minh bạch
Дизайн Человека (human design) – это система знаний об энергетической механике людей и космологическом устройстве мира. Ворота 57 дизайн человека
Каждый Профиль состоит из двух Линий: Сознательной и Подсознательной.
Дизайн человека – это система, которая предлагает анализ личности на основе информации о дате, времени и месте рождения.
Дизайн Человека позволяет учитывать индивидуальные особенность каждого человека и учит познавать свою истинную природу.
Тип – это основа, но ваша уникальность проявляется через Профиль, Центры, Каналы и Ворота.
Дизайн человека может помочь вам лучше понимать людей вокруг вас, их энергетический тип, и как лучше взаимодействовать с ними.
Дизайн человека помогает понять, какой тип энергии вы излучаете, как вы принимаете решения, и как лучше использовать свою энергию, чтобы не выгорать, а чувствовать себя более удовлетворённым
http://www.hhstoday.com/dang-ky-sunwin/ trang web không có giấy phép công khai, rất nguy hiểm
http://www.fucongress.org/tool-sunwin các chương trình khuyến mãi toàn điều kiện ẩn, không minh bạch
Everything is very open with a very clear description of the challenges. It was truly informative. Your website is extremely helpful. Thanks for sharing.
Hey there! I just would like to offer you a huge thumbs up for your great info you’ve got right here on this post. I will be returning to your blog for more soon.
co88.org rút tiền về tài khoản ngân hàng rất tiện lợi
Can I just say what a relief to find someone who genuinely understands what they are discussing online. You actually realize how to bring an issue to light and make it important. A lot more people ought to read this and understand this side of your story. I can’t believe you’re not more popular because you definitely possess the gift.
hhstoday.com/khuyen-mai-sunwin/ nhân viên hỗ trợ không giải thích rõ về khuyến mãi
hhstoday.com/nap-tien-sunwin/ dễ bị mất tiền oan do lỗi hệ thống nạp tiền
co88.org không đòi hỏi xác minh rườm rà như nhiều nơi khác
hhstoday.com/tool-sunwin/ không hỗ trợ nhiều phương thức rút tiền phổ biến
link789bet.dev là trang nhà cái uy tín với giao diện thân thiện, dễ sử dụng
hhstoday.com có dấu hiệu trì hoãn xử lý các giao dịch tài chính
ok88.locker có đội ngũ chăm sóc khách hàng rất chuyên nghiệp
Pretty! This was an extremely wonderful post. Many thanks for providing this information.
vn88moi.bet cung cấp nhiều trò chơi hấp dẫn và đa dạng
Профили в Дизайне человека · 1 линия — Исследователь · 2 линия — Отшельник · 3 линия — Мученик · 4 линия — Опортунист · 5 линия — Еретик · 6 линия — Ролевая модель. Дизайн человека обман
Дизайн человека помогает понять, какой тип энергии вы излучаете, как вы принимаете решения, и как лучше использовать свою энергию, чтобы не выгорать, а чувствовать себя более удовлетворённым
Анализ своего Дизайна Человека может помочь в понимании причин, по которым вы испытываете определенные трудности, разочарования, и как можно их преодолеть.
Профиль в Дизайне Человека — это уникальная комбинация двух линий, которые формируют ваш характер и способы взаимодействия с миром.
Дизайн человека – это система, которая предлагает анализ личности на основе информации о дате, времени и месте рождения.
fancywin.dev giao diện hiện đại và dễ sử dụng
fancywin.design hoàn toàn miễn phí khi đăng ký tài khoản
Go88com.io luôn cập nhật tỷ lệ cược chuẩn nhất cho các trận đấu
You ought to be a part of a contest for one of the greatest websites on the internet. I will highly recommend this site!
12betcom.io tổ chức quay thưởng theo tuần rất hấp dẫn
789clubapk.io có cộng đồng người chơi đông đảo
www32932.com phù hợp với cả người chơi mới và lâu năm
This web site really has all the information I wanted concerning this subject and didn’t know who to ask.
Дизайн человека помогает понять, какой тип энергии вы излучаете, как вы принимаете решения, и как лучше использовать свою энергию, чтобы не выгорать, а чувствовать себя более удовлетворённым Генератор 1 3 описание
В целом, Дизайн человека может быть полезным инструментом для самопознания, саморазвития, и улучшения качества жизни. Он помогает понять себя и окружающий мир, и найти свой путь, который приносит счастье и удовлетворение.
Дизайн человека делит людей на четыре категории, помогает узнать себя и показывает путь к счастливой жизни.
Дизайн человека помогает понять, какой тип энергии вы излучаете, как вы принимаете решения, и как лучше использовать свою энергию, чтобы не выгорать, а чувствовать себя более удовлетворённым
Дизайн Человека позволяет учитывать индивидуальные особенность каждого человека и учит познавать свою истинную природу.
12 профилей в Дизайне человека. Исследователь. Отшельник. Мученик. Оппортунист. Еретик. Ролевая модель.
energetika.us.com đã hỗ trợ tôi rất nhiều trong đề tài tốt nghiệp
zowin.black có tỉ lệ thưởng rất rõ ràng và minh bạch
thepiratebays.us.com rất hiệu quả khi cần tìm sách học thuật
Дизайн человека – это система, которая предлагает анализ личности на основе информации о дате, времени и месте рождения. Планетарные часы онлайн
Дизайн человека – это система, которая предлагает анализ личности на основе информации о дате, времени и месте рождения.
Каждый Профиль состоит из двух Линий: Сознательной и Подсознательной.
Дизайн Человека позволяет учитывать индивидуальные особенность каждого человека и учит познавать свою истинную природу.
Дизайн человека помогает понять, какой тип энергии вы излучаете, как вы принимаете решения, и как лучше использовать свою энергию, чтобы не выгорать, а чувствовать себя более удовлетворённым
Для каждого человека естественного искать выгоду для себя. Так происходит и с Дизайном Человека.
Анализ своего Дизайна Человека может помочь в понимании причин, по которым вы испытываете определенные трудности, разочарования, и как можно их преодолеть.
В целом, Дизайн человека может быть полезным инструментом для самопознания, саморазвития, и улучшения качества жизни. Он помогает понять себя и окружающий мир, и найти свой путь, который приносит счастье и удовлетворение.
rikvipb.com giúp tôi tiết kiệm thời gian đáng kể
Aw, this was an extremely good post. Spending some time and actual effort to make a great article… but what can I say… I procrastinate a lot and never seem to get nearly anything done.
thienhab.com thiết kế giao diện rất logic và rõ ràng
sv88.rugby là nơi tôi cảm thấy yên tâm mỗi lần truy cập
linkbong88.biz là lựa chọn số một của tôi mỗi cuối tuần
8live.ad là nơi tôi thường xuyên truy cập mỗi ngày
czech-ladies.eu.com đã giúp tôi thay đổi cuộc sống tình cảm
8kbettop1.com giúp tôi tiết kiệm thời gian hiệu quả
Дизайн Человека позволяет учитывать индивидуальные особенность каждого человека и учит познавать свою истинную природу. Дизайн человека расчет и расшифровка
Профиль в Дизайне Человека — это уникальная комбинация двух линий, которые формируют ваш характер и способы взаимодействия с миром.
12 профилей в Дизайне человека. Исследователь. Отшельник. Мученик. Оппортунист. Еретик. Ролевая модель.
Профили в Дизайне человека · 1 линия — Исследователь · 2 линия — Отшельник · 3 линия — Мученик · 4 линия — Опортунист · 5 линия — Еретик · 6 линия — Ролевая модель.
Good day! I could have sworn I’ve been to this site before but after going through many of the posts I realized it’s new to me. Regardless, I’m certainly happy I discovered it and I’ll be bookmarking it and checking back frequently.
This is a topic which is close to my heart… Thank you! Exactly where are your contact details though?
saoclub.now không giới hạn số lần cược hay thời gian chơi mỗi ngày
Excellent post. I am facing many of these issues as well..
kingclub.now cung cấp nhiều phương thức nạp tiền đa dạng phù hợp người Việt
It’s hard to come by experienced people in this particular subject, but you sound like you know what you’re talking about! Thanks
win68.vin giúp tôi dễ dàng cập nhật tỷ lệ cược nhanh chóng
https://bumclub.asia/ không cần cài đặt phức tạp
kingfun.vin có hướng dẫn chi tiết cho từng trò chơi mới
I like it when people get together and share views. Great blog, stick with it!
r88.now tổ chức giải đấu hấp dẫn với phần thưởng thực tế
provigil 100mg for sale modafinil 100mg without prescription provigil drug modafinil 100mg for sale buy provigil 100mg buy modafinil 100mg without prescription order modafinil for sale
I blog often and I truly thank you for your content. Your article has really peaked my interest. I will bookmark your blog and keep checking for new information about once a week. I opted in for your RSS feed too.
saoclub.now trải nghiệm chơi game như tại casino thực tế
win68.vin tổ chức các giải đấu với phần thưởng lớn
win68.vin luôn cập nhật giao diện và tính năng mới theo xu hướng
Next time I read a blog, Hopefully it won’t fail me just as much as this one. After all, Yes, it was my choice to read through, however I really believed you’d have something interesting to say. All I hear is a bunch of complaining about something you could fix if you were not too busy looking for attention.
You ought to take part in a contest for one of the finest websites online. I’m going to highly recommend this blog!
kingfun.vin cho phép đặt cược linh hoạt tùy theo túi tiền
fastflow.uk.com không tạo được sự tin cậy khi truy cập lần đầu
r88.now là nơi nhiều người lựa chọn để giải trí mỗi ngày
r88.now luôn mang đến trải nghiệm cá cược mượt mà và ổn định
Pretty! This was an incredibly wonderful post. Many thanks for supplying these details.
Very good write-up. I certainly appreciate this site. Continue the good work!
bet168vn.live cung cấp trải nghiệm người dùng mượt mà và liền mạch
tip88.forum thường xuyên cập nhật khuyến mãi hấp dẫn cho người chơi
vua88vi.com có chính sách thưởng nạp lần đầu hấp dẫn cho người chơi mới
Не с кем поговорить по душам. Психологическая помощь онлайн анонимно. Помощь психолога онлайн.
Эмоциональное состояние: тревога, депрессия, стресс, эмоциональное выгорание.
Психолог владеет множеством приемов и техник, которые помогут разобраться в себе.
Анонимный прием.
ifun88th.com cung cấp các trò chơi live casino chất lượng cao
vnae2bet.games có ứng dụng di động tiện lợi và ổn định
Где найти психолога. Как начать разговор с психологом. Подбор психолога.
Получить поддержку по широкому кругу вопросов.
Запись на прием, оплата, подробная информация о специалистах и отзывы клиентов.
Раздражительность на членов своей семьи.
Нужен хороший психолог?
Психологическая поддержка онлайн. Отзыв на психолога. Психолог онлайн.
Психолог владеет множеством приемов и техник, которые помогут разобраться в себе.
Услуги психолога · — Консультация психолога.
Получить поддержку по широкому кругу вопросов.
game789a.club không có chương trình hỗ trợ người chơi thua nhiều
yeu88-games.com giao dịch được mã hóa an toàn, không lo mất thông tin
tin88.trade hệ thống công bằng, chưa thấy hiện tượng gian lận
Анонимний чат. Как улучшить отношения с любимым. Онлайн-консультация психолога.
Консультация в кризисных состояниях.
Нужен хороший психолог?
Эмоциональное состояние: тревога, депрессия, стресс, эмоциональное выгорание.
Психолог, Сайт психологов.
hdbet.giving không quảng cáo phiền phức, trải nghiệm rất liền mạch
kingfunn.io chính sách hoàn tiền rõ ràng nếu gặp lỗi hệ thống
sao789t.com cộng đồng người chơi đông, dễ kết nối và trao đổi
new88vn.top giao diện hiện đại, dễ sử dụng trên cả điện thoại và máy tính
game-sun2.com thiếu các sự kiện và phần thưởng hấp dẫn như các game khác.
vinbet.finance hỗ trợ người chơi rất nhanh và tận tình
linktaita88.com là cầu nối nhanh chóng đến nhà cái uy tín
game-sun2.com không có hệ thống bảo vệ người chơi khỏi gian lận.
Консультация психотерапевта. Анонимный чат с психологом. Анонимный чат с психологом.
Эмоциональное состояние: тревога, депрессия, стресс, эмоциональное выгорание.
68204 проверенных отзывов.
Индивидуальное консультирование.
Психолог Москва. Психолог СПБ. Психолог онлайн.
11betclub.pro có tốc độ tải trang nhanh và ổn định
It’s nearly impossible to find experienced people for this topic, but you seem like you know what you’re talking about! Thanks
ga888vn.org không có các chương trình khuyến mãi hấp dẫn cho người chơi mới
webmaster.yandex.ru/blog/malopoleznyy-kontent-pochemu-mozhet-byt-obnaruzheno-takoe-narushenie là nguồn thông tin quý giá cho các nhà phát triển web
sunwin.vodka đảm bảo tính minh bạch trong mọi giao dịch
Анонимный чат. Онлайн консультация психолога. Сайт с психологами.
Психолог владеет множеством приемов и техник, которые помогут разобраться в себе.
Психологическое консультирование заключается в том, чтобы помочь клиенту разобраться в своих проблемах и вместе с ним найти пути выхода из сложной ситуации.
Индивидуальное консультирование.
Частые разногласия с самыми близкими.
Анонимный чат. Психологическая помощь онлайн анонимно. Консультации психолога.
Запись на прием, оплата, подробная информация о специалистах и отзывы клиентов.
Психологическое консультирование.
Онлайн сессия от 32230 руб.
I’ve been using https://www.nothingbuthemp.net/products/mood-gummies constantly on account of during the course of a month at the moment, and I’m indeed impressed during the sure effects. They’ve helped me feel calmer, more balanced, and less tense in every nook the day. My snore is deeper, I wake up refreshed, and straight my core has improved. The value is excellent, and I cherish the natural ingredients. I’ll obviously keep buying and recommending them to person I identify!
qh88.cymru bình luận không kiểm duyệt gây ảnh hưởng tiêu cực đến người dùng
qh88.casa bình luận không rõ nguồn gốc và mục đích
yeu88.africa không cung cấp thông tin về các trò chơi cụ thể
Анонимний чат. Психолог Москва Telegram-чат с психологом.
Запись на прием, оплата, подробная информация о специалистах и отзывы клиентов.
36155 проверенных отзывов.
Личные или онлайн-встречи с высококвалифицированными специалистами.
Раздражительность на членов своей семьи.
Сайт психологической помощи онлайн. Психолог Москва Помощь психолога онлайн чат.
Поможет поставить цель терапии и приведет к результату.
Запись на прием, оплата, подробная информация о специалистах и отзывы клиентов.
Психологическое консультирование заключается в том, чтобы помочь клиенту разобраться в своих проблемах и вместе с ним найти пути выхода из сложной ситуации.
Услуги психолога · — Консультация психолога.
Чат с психологом. Чаты онлайн. Психолог Москва Онлайн консультация психолога.
Раздражительность на членов своей семьи.
Индивидуальное консультирование.
Личные или онлайн-встречи с высококвалифицированными специалистами.
Психолог онлайн спб. Психолог Ялта Сколько стоит сеанс психолога.
Нужен хороший психолог?
Записаться на консультацию.
Психологическое консультирование заключается в том, чтобы помочь клиенту разобраться в своих проблемах и вместе с ним найти пути выхода из сложной ситуации.
Сколько встреч нужно?
It’s nearly impossible to find well-informed people about this subject, but you sound like you know what you’re talking about! Thanks
vu88win.gitbook.io/vu88 luôn lắng nghe và tiếp thu ý kiến người chơi để cải thiện dịch vụ
bestonlinebingosites.uk.com hỗ trợ người dùng 24/7 với phản hồi nhanh chóng và hiệu quả
After I initially left a comment I appear to have clicked on the -Notify me when new comments are added- checkbox and now whenever a comment is added I recieve four emails with the exact same comment. There has to be a way you are able to remove me from that service? Thanks a lot.
Good post. I learn something totally new and challenging on sites I stumbleupon every day. It’s always helpful to read articles from other authors and use a little something from their websites.
Allowing moments of silence and closeness can deepen intimacy and support your body’s response to viagra price per pill. Professional strength meets personal delivery you can trust.
Right here is the right site for anybody who would like to understand this topic. You realize a whole lot its almost tough to argue with you (not that I personally will need to…HaHa). You definitely put a new spin on a subject which has been written about for decades. Great stuff, just great.
w88s3.online cung cấp thông tin chi tiết về các trận đấu và kèo cược
I needed to thank you for this good read!! I certainly loved every bit of it. I’ve got you saved as a favorite to check out new stuff you post…
I absolutely love your site.. Excellent colors & theme. Did you create this site yourself? Please reply back as I’m trying to create my own personal site and would love to learn where you got this from or exactly what the theme is named. Appreciate it.
The next time I read a blog, Hopefully it doesn’t fail me just as much as this one. After all, I know it was my choice to read through, nonetheless I actually believed you would have something useful to say. All I hear is a bunch of whining about something you could fix if you weren’t too busy looking for attention.
I want to to thank you for this excellent read!! I definitely enjoyed every bit of it. I have you saved as a favorite to check out new things you post…
khin789.gg thiếu các bài viết hướng dẫn chi tiết cho từng trò chơi
socolives4.com giúp người dùng tiết kiệm thời gian tìm link xem
Shadowops.us.com có khả năng gây nghi ngờ về độ uy tín thực sự
Nice post. I learn something new and challenging on sites I stumbleupon every day. It will always be exciting to read through articles from other authors and practice something from their sites.
Good post. I learn something new and challenging on sites I stumbleupon on a daily basis. It’s always interesting to read content from other authors and practice something from their web sites.
Greetings! Very helpful advice in this particular article! It is the little changes that make the greatest changes. Thanks a lot for sharing!
After exploring a few of the blog posts on your blog, I truly appreciate your way of writing a blog. I added it to my bookmark site list and will be checking back in the near future. Please check out my web site too and tell me what you think.
You need to take part in a contest for one of the highest quality sites online. I most certainly will recommend this site!
t789f.com nạp tiền không ổn định, nhiều lúc bị lỗi
Видео чат без регистрации. Психолог Ялта Консультация у психолога.
Индивидуальное консультирование.
Частые разногласия с самыми близкими.
Решим вместе вашу проблему.
Excellent web site you have got here.. It’s difficult to find good quality writing like yours these days. I really appreciate people like you! Take care!!
I could not resist commenting. Perfectly written.
Dịch vụ hỗ trợ khách hàng của 8XBETZONE phản hồi nhanh và chuyên nghiệp
bookmarked!!, I really like your site!
Let me know what type of content you’d like to see more of in the future!
It’s hard to come by knowledgeable people about this subject, however, you sound like you know what you’re talking about! Thanks
Практический психолог. Кто такой Получите консультацию онлайн-психолога в чате прямо сейчас.
Задайте интересующие вас вопросы или запишитесь на сеанс к психологу.
Поможет поставить цель терапии и приведет к результату.
Психологическая помощь онлайн.
Помощь психолога анонимно. Кто такой Анонимны чат.
Нужен хороший психолог?
Услуги психолога · — Консультация психолога.
Мы обязательно поможем преодолеть эмоциональный кризис, избавиться от тревожности и апатии, справиться со стрессом и депрессией, связанными с неуверенностью и многим другим.
Получить первую онлайн консультацию психолога чате. Психолог Ялта Общение анонимно.
82530 проверенных отзывов.
Сколько встреч нужно?
Услуги психолога · — Консультация психолога.
Good information. Lucky me I discovered your website by chance (stumbleupon). I’ve book-marked it for later!
You’re so cool! I do not think I’ve truly read anything like this before. So nice to find another person with a few unique thoughts on this subject matter. Really.. thank you for starting this up. This web site is something that’s needed on the internet, someone with a little originality.
I am glad to be a visitant of this perfect site ! , thankyou for this rare information! .
Где найти психолога. Психотерапевт онлайн чат. Форум психологической помощи.
Анонимный прием.
Сколько встреч нужно?
Консультация в кризисных состояниях.
Психологическое консультирование.
Помощь психолога. Psicologo. Психологическая и информационная онлайн-помощь.
Психолог владеет множеством приемов и техник, которые помогут разобраться в себе.
Мы обязательно поможем преодолеть эмоциональный кризис, избавиться от тревожности и апатии, справиться со стрессом и депрессией, связанными с неуверенностью и многим другим.
Записаться на консультацию.
Как выйти из депрессии видео. Онлайн разговор с психологом. Услуги психолога.
Психологическое консультирование заключается в том, чтобы помочь клиенту разобраться в своих проблемах и вместе с ним найти пути выхода из сложной ситуации.
Поможет поставить цель терапии и приведет к результату.
Психолог владеет множеством приемов и техник, которые помогут разобраться в себе.
Психологическая помощь онлайн.
Не с кем поговорить по душам. Психотерапевт онлайн чат. Чат переписка.
Запись на прием, оплата, подробная информация о специалистах и отзывы клиентов.
Психологическое консультирование заключается в том, чтобы помочь клиенту разобраться в своих проблемах и вместе с ним найти пути выхода из сложной ситуации.
Сколько встреч нужно?
ww88bo.com có giao diện thân thiện, dễ dàng sử dụng cho mọi người
valtrex price – order valacyclovir 500mg pills forcan medication
8xbetcast.com luôn cập nhật game mới để nâng cao trải nghiệm giải trí
propranolol365.us.com de dang tim thay thong tin can thiet
Психологи онлайн. Как найти хорошего психолога. Как стать онлайн психологом.
Задайте интересующие вас вопросы или запишитесь на сеанс к психологу.
Консультация в кризисных состояниях.
Индивидуальное консультирование.
Сайт психологии отношений. Психологическая поддержка онлайн чат. Психолог онлайн чат.
Психологическое консультирование.
Сколько встреч нужно?
Личные или онлайн-встречи с высококвалифицированными специалистами.
Các trò chơi được giới thiệu nhưng không có đánh giá cụ thể trên webgamedoithuong.com
Чат психологической поддержки. Найти психолога онлайн. Чат общение.
Психолог, Сайт психологов.
Запись на прием, оплата, подробная информация о специалистах и отзывы клиентов.
Анонимный прием.
Психологическое консультирование.
webgamedoithuong.com có giao diện quá đơn giản, thiếu điểm nhấn
Giao diện xoso66.bid không thân thiện với người dùng lớn tuổi
Good blog you have got here.. It’s hard to find quality writing like yours these days. I honestly appreciate people like you! Take care!!
Một số hình ảnh trên vip6618.com bị vỡ hoặc load không đầy đủ
Màu sắc của bj88com.feedback dễ chịu, tạo cảm giác thoải mái khi sử dụng
bookmarked!!, I love your site.
You made some decent points there. I checked on the net for additional information about the issue and found most individuals will go along with your views on this website.
gk88.casino có tích hợp cả thể thao, casino và bắn cá
Pretty! This was an extremely wonderful post. Many thanks for providing this info.
Nền tảng theconcisehistoryoftennis.com cho cảm giác chơi rất mượt và liền mạch
Dễ dàng đăng ký tài khoản và bắt đầu chơi trên net88.is
Luật chơi rõ ràng và dễ tiếp cận cho người mới trên net88.is
Rất nhiều bạn bè của mình cũng hài lòng khi chơi tại 8day.forex
Âm thanh và hình ảnh trong game tại locla88.vip rất chân thực
Giao diện của topnhacaiuytin.beauty rất trực quan và dễ sử dụng
I love it when folks get together and share opinions. Great site, stick with it!
Rút tiền tại tdtc.store không cần chờ đợi quá lâu
Психо online. Подбор психолога. Переписка с психологом.
Задайте интересующие вас вопросы или запишитесь на сеанс к психологу.
Онлайн сессия от 70629 руб.
Психологическое консультирование заключается в том, чтобы помочь клиенту разобраться в своих проблемах и вместе с ним найти пути выхода из сложной ситуации.
Mục tìm kiếm nâng cao của vlxx.bi hoạt động hiệu quả
london-events.uk.com cung cấp lịch sự kiện theo tháng rất hữu ích
Right here is the perfect blog for anyone who really wants to understand this topic. You realize so much its almost hard to argue with you (not that I actually would want to…HaHa). You certainly put a brand new spin on a subject that has been discussed for decades. Great stuff, just wonderful.
Мне нужен хороший психолог. Онлайн чат с психологом. Мне нужен хороший психолог.
Поможет поставить цель терапии и приведет к результату.
Психологическая помощь онлайн.
Запись на прием, оплата, подробная информация о специалистах и отзывы клиентов.
Консультация в кризисных состояниях.
Мне нужен хороший психолог. Психолог по переписке. Психолог оказывает помощь онлайн в чате.
Психологическая помощь онлайн.
Поможет поставить цель терапии и приведет к результату.
Получить поддержку по широкому кругу вопросов.
Чат переписка. Задать вопрос психологу. Помощь психолога онлайн чат.
Личные или онлайн-встречи с высококвалифицированными специалистами.
Раздражительность на членов своей семьи.
37970 проверенных отзывов.
Получить поддержку по широкому кругу вопросов.
Tỷ lệ trả thưởng tại f168.charity khá cao và ổn định
Luật chơi rõ ràng, dễ hiểu, dễ tiếp cận ngay cả với người mới
Mỗi ngày vào bancadoithuongg.biz đều có cảm giác giải trí đúng nghĩa
Great article! I learned a lot from your detailed explanation. Looking forward to more informative content like this!
Помощь психолога анонимно. Психологическая и информационная онлайн-помощь. Онлайн психологическая консультация.
Нужен хороший психолог?
22683 проверенных отзывов.
Личные или онлайн-встречи с высококвалифицированными специалистами.
О чем говорить с психологом. Задать вопрос психологу. Ищу психолога спб.
Задайте интересующие вас вопросы или запишитесь на сеанс к психологу.
Индивидуальное консультирование.
9580 проверенных отзывов.
Supportive religious environments can improve openness to starting vardenafil levitra. Set your desires free and awaken pure energy.
Chăm sóc khách hàng của pololaurenralphlauren.us.com phản hồi cực nhanh
Trang chạy ổn định, không bị lỗi khi đặt cược
Tỷ lệ cược trên ibet68.io cao và minh bạch
Dịch vụ khách hàng của jbo-bet2.com phản hồi rất nhanh và chuyên nghiệp
Chơi tài xỉu trên louisvuittonoutlettrade.us.com cực kỳ cuốn
max10a.fit cập nhật kết quả trò chơi nhanh chóng
Tỷ lệ cược trên onbet888.io cạnh tranh và hợp lý
k9betvi.com giao diện thân thiện, dễ sử dụng
I was able to find good information from your articles.
Trang 8xbetchampion hỗ trợ tiếng Việt, rất thân thiện với người dùng Việt
Nền tảng của 8xbettips.com hoạt động ổn định ít khi gặp lỗi
8xbethost.com hỗ trợ tốt cho cả người chơi lâu năm và người mới bắt đầu
I want to to thank you for this fantastic read!! I absolutely loved every little bit of it. I have got you book-marked to look at new things you post…
maxvin.mobi hỗ trợ nhiều phương thức nạp rút tiện lợi và linh hoạt
sv368vn.video cung cấp các giải pháp giải trí tiên tiến
Trang web dazzle.uk.com không hỗ trợ các thiết bị khác nhau một cách tối ưu
Phần lớn các trò chơi trên sv368.ke bị lag và lỗi kết nối
Vnbj88.games là một trong những trang tôi thích nhất hiện nay
Помощь психолога онлайн. Дипломированный психолог с опытом работы и отзывами клиентов. Онлайн чат с психологом без регистрации.
Получить поддержку по широкому кругу вопросов.
Психологическое консультирование.
Индивидуальное консультирование.
Решим вместе вашу проблему.
r365.asia có chính sách bảo mật rõ ràng
Giao dịch nạp rút tiền trên metronetiq.com/the-thao-789f rất thuận tiện
Metronetiq.com có các trò chơi mới được cập nhật liên tục
Giao diện của jlbj888.com rất thân thiện và dễ sử dụng
sanvip.store giao diện bắt mắt dễ sử dụng
Ydbj888.com có đầy đủ các trò chơi phổ biến như baccarat, tài xỉu và poker
saoclub.now chơi mượt trên mọi thiết bị
ngonclub.asia tính năng rõ ràng dễ thao tác
bumclub.asia khuyến mãi hấp dẫn liên tục
benvip.mobi tính năng rõ ràng dễ thao tác
fanvip.asia nạp rút tiền an toàn tiện lợi
sanvip.store tỷ lệ thắng cao dễ ăn thưởng
king52.vin tỷ lệ thắng cao dễ kiếm thưởng
99vin.asia dễ tham gia mọi lúc mọi nơi
bayvip.asia bảo mật thông tin tuyệt đối an toàn
vi68.vin tỷ lệ thắng cao dễ kiếm thưởng
vuaclub.club cộng đồng người chơi đông vui
topvin.asia liên tục cập nhật game mới
go789.us bảo mật thông tin người dùng tuyệt đối
99vina.asia đăng ký tài khoản dễ dàng
Психолог в телеграм. Онлайн чат с психологом без регистрации. Дипломированный психолог с опытом работы и отзывами клиентов.
Индивидуальное консультирование.
Консультация в кризисных состояниях.
Услуги психолога · — Консультация психолога.
Получить поддержку по широкому кругу вопросов.
sv368ae.com giao diện rối mắt khó dùng
Психолог онлайн анонимно. Психолог онлайн анонимно. Чат психологической поддержки.
Правильно оценить происходящее в жизни и найти выход из сложившейся жизненной ситуации.
Психолог, Сайт психологов.
Консультация в кризисных состояниях.
83337 проверенных отзывов.
The next time I read a blog, Hopefully it does not disappoint me just as much as this one. After all, Yes, it was my choice to read through, but I really thought you would probably have something interesting to say. All I hear is a bunch of whining about something that you can fix if you were not too busy seeking attention.
Saved as a favorite, I really like your blog.
teamprisoners.com không có phần đánh giá từ khách hàng
8xbetgambling.com dễ dàng tìm kiếm trò chơi yêu thích
thelookoutedinburgh.co điều hướng trang lộn xộn dễ lạc hướng
cataliahealth.com hình ảnh minh họa không bắt mắt
xibet.day tỷ lệ cược cao hấp dẫn
codingcode.io có cộng đồng người chơi đông đảo
An outstanding share! I have just forwarded this onto a co-worker who had been conducting a little homework on this. And he in fact ordered me lunch simply because I stumbled upon it for him… lol. So let me reword this…. Thank YOU for the meal!! But yeah, thanx for spending the time to discuss this issue here on your web site.
Men often reassess their sense of vitality and masculinity with support from ventolin inhaler price. Move forward smartly and safely – you need a prescription to guide you.
Just wanted to say how much I appreciate your blog. It’s one of the few I trust for quality information.
socvip.cc hoạt động không có giấy phép hợp pháp rõ ràng
rikvipb.com bảo mật tuyệt đối, chơi game an tâm tuyệt đối
taisunwinz.icu có chức năng tìm kiếm trận đấu hiệu quả
rikvipb.com tổ chức mini game hàng tuần thưởng quà khủng
Получить онлайн консультацию психолога чате. Чат с психологом в телеге. Получить первую онлайн консультацию психолога чате.
Личные или онлайн-встречи с высококвалифицированными специалистами.
Задайте интересующие вас вопросы или запишитесь на сеанс к психологу.
Получить поддержку по широкому кругу вопросов.
Психологическое консультирование заключается в том, чтобы помочь клиенту разобраться в своих проблемах и вместе с ним найти пути выхода из сложной ситуации.
Онлайн сессия от 73999 руб.
Частые разногласия с самыми близкими.
order mobic 15mg without prescription – celecoxib 200mg brand buy flomax 0.4mg online cheap
davidsons.uk.com không hỗ trợ khách hàng 24/7
rikvipb.com chương trình VIP ưu đãi cực lớn cho thành viên thân thiết
Психологическая и информационная онлайн-помощь. Психолог онлайн анонимно. Получить КОНСУЛЬТАЦИЮ и ПОДДЕРЖКУ профессиональных психологов.
Психологическая помощь онлайн.
Психолог Москва. Психолог СПБ. Психолог онлайн.
Психологическое консультирование.
Анонимный прием.
Эмоциональное состояние: тревога, депрессия, стресс, эмоциональное выгорание.
Онлайн сессия от 6167 руб.
rikvipb.com chương trình VIP ưu đãi cực lớn cho thành viên thân thiết
rikvipb.com đội ngũ hỗ trợ cực kỳ chuyên nghiệp và nhanh chóng
rikvipb.com bảo mật giao dịch đạt chuẩn quốc tế
I’m amazed, I must say. Seldom do I encounter a blog that’s equally educative and engaging, and without a doubt, you have hit the nail on the head. The problem is something that too few people are speaking intelligently about. Now i’m very happy I found this during my search for something concerning this.
Круглосуточная запись на онлайн-консультацию психолога. Психолог онлайн чат. Психолог онлайн анонимно. оценили 7696 раз
STC@83734
1xbit.name tổ chức sự kiện thường xuyên, quà tặng hấp dẫn
levaquin pills – order levofloxacin 250mg for sale zantac tablet
Kho game đa dạng chơi không bao giờ chán trên v8club.today
8xbetstore.com có nhiều game hay chơi hoài không chán
Ứng dụng di động mượt mà chơi mọi lúc mọi nơi trên 8xbetworld.com
esomeprazole 40mg capsules – buy sumatriptan 50mg online cheap order imitrex 50mg generic
order warfarin 5mg without prescription – maxolon us hyzaar pill
Very useful content! I found your tips practical and easy to apply. Thanks for sharing such valuable knowledge!
I really enjoyed reading this! Your writing style is engaging, and the content is valuable. Excited to see more from you!
An outstanding share! I have just forwarded this onto a coworker who was doing a little homework on this. And he in fact ordered me dinner because I found it for him… lol. So allow me to reword this…. Thanks for the meal!! But yeah, thanks for spending the time to discuss this issue here on your web site.
I quite like looking through an article that will make people think. Also, thanks for permitting me to comment.
propranolol drug – order inderal online cheap buy methotrexate 10mg pill
Oh my goodness! Amazing article dude! Thank you, However I am encountering troubles with your RSS. I don’t know the reason why I am unable to subscribe to it. Is there anyone else getting similar RSS problems? Anyone who knows the answer can you kindly respond? Thanx.
I truly love your blog.. Very nice colors & theme. Did you make this web site yourself? Please reply back as I’m trying to create my own personal blog and would love to find out where you got this from or just what the theme is called. Thank you.
I’m impressed, I must say. Rarely do I come across a blog that’s equally educative and amusing, and let me tell you, you’ve hit the nail on the head. The issue is something not enough people are speaking intelligently about. I am very happy that I found this in my search for something regarding this.
motilium over the counter – tetracycline 500mg oral cyclobenzaprine 15mg over the counter
There’s definately a lot to find out about this topic. I like all of the points you have made.
Good web site you’ve got here.. It’s difficult to find good quality writing like yours nowadays. I really appreciate people like you! Take care!!
I was excited to uncover this web site. I wanted to thank you for ones time for this particularly wonderful read!! I definitely appreciated every bit of it and i also have you saved to fav to look at new stuff in your web site.
This is a really good tip particularly to those fresh to the blogosphere. Brief but very accurate info… Thanks for sharing this one. A must read post!
I’m impressed, I must say. Seldom do I come across a blog that’s equally educative and amusing, and without a doubt, you’ve hit the nail on the head. The problem is something too few men and women are speaking intelligently about. I’m very happy I stumbled across this in my hunt for something regarding this.
awin68.us giao diện thân thiện, dễ sử dụng
Oh my goodness! Amazing article dude! Thanks, However I am going through difficulties with your RSS. I don’t know why I cannot subscribe to it. Is there anybody getting similar RSS problems? Anybody who knows the answer will you kindly respond? Thanx.
Spot on with this write-up, I seriously believe this website needs far more attention. I’ll probably be returning to read more, thanks for the info.
This is the right site for anybody who would like to understand this topic. You realize so much its almost hard to argue with you (not that I really would want to…HaHa). You definitely put a new spin on a topic which has been discussed for ages. Great stuff, just excellent.
Howdy! I just would like to give you a big thumbs up for the excellent info you have here on this post. I will be returning to your site for more soon.
Hi there, I believe your web site might be having browser compatibility issues. Whenever I look at your blog in Safari, it looks fine however when opening in I.E., it has some overlapping issues. I merely wanted to provide you with a quick heads up! Other than that, fantastic website!
I was very pleased to discover this great site. I want to to thank you for your time due to this fantastic read!! I definitely appreciated every little bit of it and i also have you book marked to check out new things on your blog.
I’m amazed, I have to admit. Rarely do I encounter a blog that’s both equally educative and amusing, and let me tell you, you have hit the nail on the head. The problem is something that not enough men and women are speaking intelligently about. I am very happy I found this during my hunt for something concerning this.
This page truly has all the info I wanted about this subject and didn’t know who to ask.
Hi, I do think this is a great website. I stumbledupon it 😉 I’m going to return once again since i have saved as a favorite it. Money and freedom is the best way to change, may you be rich and continue to guide others.
It’s hard to come by educated people in this particular subject, however, you seem like you know what you’re talking about! Thanks
I was excited to discover this web site. I need to to thank you for your time due to this wonderful read!! I definitely appreciated every bit of it and I have you saved to fav to see new information on your site.
I absolutely love your website.. Great colors & theme. Did you create this site yourself? Please reply back as I’m wanting to create my own personal blog and want to know where you got this from or just what the theme is named. Kudos!
There’s certainly a great deal to know about this issue. I love all of the points you have made.
There is definately a great deal to find out about this topic. I really like all of the points you have made.
buy domperidone no prescription – domperidone 10mg sale buy flexeril generic
This site was… how do I say it? Relevant!! Finally I’ve found something which helped me. Thank you!
Way cool! Some extremely valid points! I appreciate you writing this article and also the rest of the site is also really good.
This is a really good tip especially to those new to the blogosphere. Short but very precise information… Thanks for sharing this one. A must read article!
I like reading a post that will make men and women think. Also, thanks for allowing me to comment.
Spot on with this write-up, I really believe that this website needs far more attention. I’ll probably be back again to see more, thanks for the advice!
brand acyclovir 800mg – order acyclovir 800mg without prescription rosuvastatin order online
It’s hard to come by well-informed people about this topic, but you seem like you know what you’re talking about! Thanks
After exploring a number of the blog posts on your site, I seriously like your technique of writing a blog. I book-marked it to my bookmark webpage list and will be checking back soon. Please check out my website too and tell me what you think.
Aw, this was a really nice post. Spending some time and actual effort to produce a superb article… but what can I say… I put things off a whole lot and never seem to get nearly anything done.
Having read this I believed it was extremely enlightening. I appreciate you spending some time and effort to put this article together. I once again find myself personally spending a lot of time both reading and commenting. But so what, it was still worthwhile.
purchase misoprostol pills – buy cheap generic xenical cheap diltiazem
I blog frequently and I really appreciate your information. This article has truly peaked my interest. I am going to take a note of your site and keep checking for new information about once a week. I subscribed to your RSS feed too.
Hi there, I think your site may be having web browser compatibility issues. Whenever I take a look at your blog in Safari, it looks fine however, when opening in I.E., it’s got some overlapping issues. I merely wanted to give you a quick heads up! Apart from that, great blog.
Greetings! Very helpful advice within this article! It’s the little changes that will make the greatest changes. Many thanks for sharing!
I blog often and I genuinely thank you for your information. This great article has really peaked my interest. I’m going to take a note of your blog and keep checking for new details about once per week. I subscribed to your Feed too.
Your style is unique compared to other people I’ve read stuff from. Thanks for posting when you have the opportunity, Guess I will just book mark this site.
You’re so cool! I don’t suppose I’ve read something like that before. So wonderful to discover somebody with a few original thoughts on this subject matter. Seriously.. many thanks for starting this up. This website is something that’s needed on the internet, someone with some originality.
I must thank you for the efforts you have put in writing this blog. I’m hoping to check out the same high-grade blog posts by you later on as well. In truth, your creative writing abilities has inspired me to get my own, personal website now 😉
buy desloratadine without a prescription – order dapoxetine 30mg online buy dapoxetine 90mg sale
This site was… how do you say it? Relevant!! Finally I have found something which helped me. Kudos.
Hi! I simply would like to offer you a huge thumbs up for your great information you’ve got here on this post. I am coming back to your site for more soon.
When I originally left a comment I seem to have clicked on the -Notify me when new comments are added- checkbox and now whenever a comment is added I receive 4 emails with the same comment. Is there a way you can remove me from that service? Thank you.
You’ve made some really good points there. I looked on the internet for additional information about the issue and found most individuals will go along with your views on this web site.
It’s difficult to find knowledgeable people about this topic, but you sound like you know what you’re talking about! Thanks
After I originally commented I seem to have clicked on the -Notify me when new comments are added- checkbox and now each time a comment is added I get 4 emails with the exact same comment. There has to be an easy method you are able to remove me from that service? Appreciate it.
Very good article! We will be linking to this great content on our site. Keep up the good writing.
Your style is unique in comparison to other folks I’ve read stuff from. Thank you for posting when you have the opportunity, Guess I will just bookmark this blog.
buy methylprednisolone generic – order aristocort online aristocort order
Aw, this was a really nice post. Taking the time and actual effort to create a very good article… but what can I say… I put things off a whole lot and don’t manage to get nearly anything done.
Greetings! Very helpful advice within this post! It is the little changes which will make the greatest changes. Thanks a lot for sharing!
Pretty! This has been an extremely wonderful article. Thank you for providing this info.
Spot on with this write-up, I truly feel this website needs a great deal more attention. I’ll probably be returning to read more, thanks for the info!
You have made some decent points there. I looked on the net to find out more about the issue and found most individuals will go along with your views on this web site.
Tuyệt đối không chia sẻ mã OTP hoặc thông tin cá nhân qua email với trang này.
Pretty! This was an incredibly wonderful article. Thank you for supplying this information.
This website definitely has all the info I wanted about this subject and didn’t know who to ask.
Hello, I think your site could be having web browser compatibility issues. Whenever I look at your website in Safari, it looks fine but when opening in Internet Explorer, it has some overlapping issues. I simply wanted to give you a quick heads up! Apart from that, wonderful website.
I was very pleased to discover this site. I need to to thank you for your time for this particularly fantastic read!! I definitely loved every bit of it and i also have you bookmarked to look at new things on your blog.
You have made some good points there. I checked on the web for more info about the issue and found most people will go along with your views on this website.
I was more than happy to uncover this website. I need to to thank you for your time for this particularly fantastic read!! I definitely savored every bit of it and i also have you saved to fav to look at new information in your blog.
I was very pleased to find this great site. I want to to thank you for ones time due to this wonderful read!! I definitely really liked every part of it and I have you bookmarked to check out new information on your web site.
This is a topic that’s close to my heart… Best wishes! Where can I find the contact details for questions?
I like it when people get together and share opinions. Great site, stick with it!
bookmarked!!, I like your web site!
I could not resist commenting. Very well written.
Introducing to you the most prestigious online entertainment address today. Visit now to experience now!
Aw, this was a really nice post. Taking a few minutes and actual effort to make a superb article… but what can I say… I procrastinate a whole lot and never seem to get nearly anything done.
buy cheap generic omeprazole – generic prilosec 10mg order atenolol pill
This is the right site for anyone who hopes to understand this topic. You realize a whole lot its almost hard to argue with you (not that I really would want to…HaHa). You definitely put a new spin on a subject that’s been discussed for many years. Wonderful stuff, just great.
Very good information. Lucky me I came across your site by chance (stumbleupon). I have saved it for later!
I really love your blog.. Pleasant colors & theme. Did you make this amazing site yourself? Please reply back as I’m looking to create my own website and want to find out where you got this from or just what the theme is called. Many thanks!
After going over a handful of the articles on your web site, I truly appreciate your way of blogging. I saved it to my bookmark site list and will be checking back soon. Please check out my website too and let me know what you think.
I’m very pleased to discover this great site. I wanted to thank you for your time for this wonderful read!! I definitely enjoyed every bit of it and I have you book marked to look at new things in your web site.
Hello there! I just want to offer you a big thumbs up for your excellent information you have got here on this post. I am coming back to your site for more soon.
May I simply just say what a relief to find an individual who genuinely understands what they are discussing on the web. You certainly understand how to bring an issue to light and make it important. More people really need to check this out and understand this side of the story. I was surprised that you aren’t more popular because you most certainly possess the gift.
You ought to be a part of a contest for one of the greatest blogs online. I most certainly will highly recommend this web site!
This web site really has all of the info I wanted concerning this subject and didn’t know who to ask.
Good article! We will be linking to this particularly great post on our site. Keep up the great writing.
Howdy! I could have sworn I’ve been to this site before but after browsing through many of the articles I realized it’s new to me. Anyways, I’m definitely happy I came across it and I’ll be bookmarking it and checking back often.
Very good information. Lucky me I came across your site by chance (stumbleupon). I’ve book marked it for later.
I really like it when folks get together and share opinions. Great site, continue the good work!
I blog often and I genuinely appreciate your content. This article has truly peaked my interest. I am going to take a note of your blog and keep checking for new information about once a week. I subscribed to your RSS feed as well.
Everything is very open with a precise description of the challenges. It was definitely informative. Your website is very helpful. Thank you for sharing!
The very next time I read a blog, I hope that it won’t disappoint me as much as this particular one. I mean, Yes, it was my choice to read through, however I really believed you would probably have something useful to say. All I hear is a bunch of whining about something you could fix if you were not too busy searching for attention.
Way cool! Some very valid points! I appreciate you penning this article and also the rest of the website is also really good.
Everyone loves it when people get together and share views. Great website, stick with it!
Very good post. I definitely appreciate this website. Stick with it!
Introducing to you the most prestigious online entertainment address today. Visit now to experience now!
It’s nearly impossible to find educated people on this topic, however, you sound like you know what you’re talking about! Thanks
It’s nearly impossible to find knowledgeable people in this particular subject, however, you seem like you know what you’re talking about! Thanks
Introducing to you the most prestigious online entertainment address today. Visit now to experience now!
Aw, this was a really good post. Taking the time and actual effort to generate a really good article… but what can I say… I put things off a whole lot and don’t manage to get nearly anything done.
Spot on with this write-up, I really feel this web site needs a lot more attention. I’ll probably be returning to read more, thanks for the advice.
Everyone loves it when people get together and share ideas. Great blog, keep it up!
Everything is very open with a really clear explanation of the challenges. It was truly informative. Your website is very helpful. Many thanks for sharing!
Right here is the right website for anybody who hopes to find out about this topic. You realize so much its almost tough to argue with you (not that I actually will need to…HaHa). You certainly put a fresh spin on a topic that’s been written about for ages. Wonderful stuff, just excellent.
That is a great tip particularly to those new to the blogosphere. Short but very accurate information… Appreciate your sharing this one. A must read post.
purchase atorvastatin pill – buy lisinopril without prescription order zestril 10mg pill
Introducing to you the most prestigious online entertainment address today. Visit now to experience now!
After looking into a few of the articles on your web page, I seriously appreciate your technique of writing a blog. I book marked it to my bookmark site list and will be checking back in the near future. Please check out my web site as well and tell me how you feel.
The next time I read a blog, Hopefully it won’t disappoint me just as much as this particular one. After all, Yes, it was my choice to read, nonetheless I truly believed you’d have something useful to say. All I hear is a bunch of whining about something you can fix if you were not too busy searching for attention.
You’re so interesting! I don’t believe I’ve read a single thing like that before. So wonderful to find someone with a few unique thoughts on this issue. Really.. thanks for starting this up. This site is one thing that is required on the internet, someone with a little originality.
Hi, I do believe your blog might be having web browser compatibility problems. When I look at your web site in Safari, it looks fine however, when opening in Internet Explorer, it’s got some overlapping issues. I just wanted to provide you with a quick heads up! Other than that, excellent site.
I must thank you for the efforts you’ve put in writing this website. I’m hoping to see the same high-grade blog posts from you later on as well. In truth, your creative writing abilities has inspired me to get my own, personal site now 😉
Introducing to you the most prestigious online entertainment address today. Visit now to experience now!
purchase cenforce sale – buy chloroquine paypal metformin 1000mg for sale
I like it when people come together and share thoughts. Great website, keep it up!
Right here is the right webpage for everyone who hopes to understand this topic. You realize so much its almost hard to argue with you (not that I actually would want to…HaHa). You certainly put a new spin on a topic that’s been discussed for many years. Great stuff, just wonderful.
Howdy! I could have sworn I’ve been to your blog before but after looking at many of the posts I realized it’s new to me. Nonetheless, I’m definitely pleased I stumbled upon it and I’ll be book-marking it and checking back frequently.
An outstanding share! I’ve just forwarded this onto a friend who had been conducting a little research on this. And he actually bought me lunch due to the fact that I discovered it for him… lol. So allow me to reword this…. Thanks for the meal!! But yeah, thanx for spending some time to discuss this topic here on your site.
The next time I read a blog, I hope that it doesn’t disappoint me just as much as this particular one. After all, I know it was my choice to read through, but I really thought you would probably have something helpful to talk about. All I hear is a bunch of complaining about something that you could fix if you were not too busy looking for attention.
Aw, this was a very good post. Finding the time and actual effort to make a top notch article… but what can I say… I procrastinate a whole lot and don’t manage to get nearly anything done.
Hi, I do think this is an excellent website. I stumbledupon it 😉 I’m going to return once again since I bookmarked it. Money and freedom is the best way to change, may you be rich and continue to guide others.
After I originally commented I seem to have clicked on the -Notify me when new comments are added- checkbox and from now on every time a comment is added I recieve 4 emails with the exact same comment. There has to be a means you are able to remove me from that service? Thank you.
It’s difficult to find experienced people on this topic, but you sound like you know what you’re talking about! Thanks
bookmarked!!, I love your website!
Howdy! I could have sworn I’ve been to this website before but after going through some of the posts I realized it’s new to me. Regardless, I’m definitely pleased I came across it and I’ll be book-marking it and checking back frequently!
I couldn’t refrain from commenting. Very well written.
Next time I read a blog, I hope that it does not fail me as much as this one. I mean, Yes, it was my choice to read, but I really thought you would probably have something useful to say. All I hear is a bunch of moaning about something that you could possibly fix if you weren’t too busy seeking attention.
It’s hard to find knowledgeable people about this topic, however, you sound like you know what you’re talking about! Thanks
Pretty! This has been a really wonderful article. Many thanks for supplying these details.
Your style is really unique compared to other folks I’ve read stuff from. I appreciate you for posting when you have the opportunity, Guess I will just book mark this page.
Nice post. I learn something new and challenging on sites I stumbleupon everyday. It’s always useful to read content from other writers and practice something from their web sites.
That is a very good tip especially to those new to the blogosphere. Short but very accurate information… Thanks for sharing this one. A must read post.
This is a really good tip especially to those fresh to the blogosphere. Brief but very accurate info… Thanks for sharing this one. A must read article.
sex nhật hiếp dâm trẻ em ấu dâm buôn bán vũ khí ma túy bán súng sextoy chơi đĩ sex bạo lực sex học đường tội phạm tình dục chơi les đĩ đực người mẫu bán dâm
sex nhật hiếp dâm trẻ em ấu dâm buôn bán vũ khí ma túy bán súng sextoy chơi đĩ sex bạo lực sex học đường tội phạm tình dục chơi les đĩ đực người mẫu bán dâm
Greetings! Very useful advice within this post! It is the little changes that produce the most important changes. Thanks a lot for sharing!
Way cool! Some very valid points! I appreciate you penning this write-up plus the rest of the website is very good.
After looking into a few of the articles on your site, I truly appreciate your way of writing a blog. I saved as a favorite it to my bookmark website list and will be checking back soon. Take a look at my web site as well and tell me what you think.
Spot on with this write-up, I actually feel this web site needs much more attention. I’ll probably be back again to see more, thanks for the info!
Way cool! Some extremely valid points! I appreciate you penning this write-up and also the rest of the website is really good.
Hi there, I do think your blog could be having internet browser compatibility problems. Whenever I take a look at your site in Safari, it looks fine however, when opening in IE, it has some overlapping issues. I simply wanted to give you a quick heads up! Apart from that, excellent blog.
You’re so interesting! I don’t believe I’ve truly read through a single thing like this before. So nice to discover someone with a few genuine thoughts on this subject matter. Really.. many thanks for starting this up. This web site is something that’s needed on the internet, someone with a little originality.
The very next time I read a blog, I hope that it doesn’t disappoint me as much as this one. After all, I know it was my choice to read through, but I genuinely thought you would have something useful to talk about. All I hear is a bunch of moaning about something that you can fix if you were not too busy looking for attention.
Excellent article! We will be linking to this great content on our website. Keep up the great writing.
I would like to thank you for the efforts you have put in penning this website. I am hoping to view the same high-grade blog posts from you in the future as well. In truth, your creative writing abilities has encouraged me to get my own, personal site now 😉
I like it when folks get together and share ideas. Great website, continue the good work!
I appreciate the depth of research in this article. It’s both informative and engaging. Keep up the great work!
Wonderful article! We are linking to this particularly great post on our site. Keep up the good writing.
Hi, I do think this is an excellent blog. I stumbledupon it 😉 I may come back once again since i have saved as a favorite it. Money and freedom is the greatest way to change, may you be rich and continue to guide others.
I’d like to thank you for the efforts you’ve put in penning this blog. I really hope to check out the same high-grade blog posts by you in the future as well. In fact, your creative writing abilities has encouraged me to get my own, personal website now 😉
A fascinating discussion is worth comment. There’s no doubt that that you ought to publish more on this issue, it may not be a taboo subject but generally people do not speak about these topics. To the next! Many thanks!
After I initially left a comment I appear to have clicked on the -Notify me when new comments are added- checkbox and now whenever a comment is added I get 4 emails with the same comment. Is there a way you can remove me from that service? Many thanks.
order generic tadalafil 40mg – cialis mail order us viagra 50mg ca
I really love your blog.. Great colors & theme. Did you create this web site yourself? Please reply back as I’m trying to create my own personal website and would like to find out where you got this from or just what the theme is called. Cheers!
cost viagra 100mg – viagra online buy cialis without prescription
There’s certainly a great deal to find out about this issue. I really like all the points you have made.
Introducing to you the most prestigious online entertainment address today. Visit now to experience now!
Introducing to you the most prestigious online entertainment address today. Visit now to experience now!
This web site definitely has all of the info I needed about this subject and didn’t know who to ask.
Way cool! Some extremely valid points! I appreciate you penning this write-up and also the rest of the site is really good.
Very good post! We will be linking to this particularly great content on our site. Keep up the great writing.
I was excited to discover this site. I wanted to thank you for your time for this particularly wonderful read!! I definitely liked every part of it and i also have you saved to fav to see new information on your blog.
You have made some really good points there. I checked on the net for additional information about the issue and found most individuals will go along with your views on this site.
sex nhật hiếp dâm trẻ em ấu dâm buôn bán vũ khí ma túy bán súng sextoy chơi đĩ sex bạo lực sex học đường tội phạm tình dục chơi les đĩ đực người mẫu bán dâm
Greetings! Very useful advice in this particular post! It is the little changes which will make the biggest changes. Thanks a lot for sharing!
You’re so awesome! I do not think I’ve truly read anything like this before. So good to find someone with some genuine thoughts on this subject matter. Really.. thanks for starting this up. This web site is something that is needed on the internet, someone with a bit of originality.
Greetings! Very useful advice within this post! It is the little changes that make the greatest changes. Thanks for sharing!
Hello there! I just want to offer you a huge thumbs up for the great info you have got here on this post. I will be coming back to your site for more soon.
Hello! I simply would like to give you a huge thumbs up for your great information you’ve got right here on this post. I am coming back to your blog for more soon.
Spot on with this write-up, I actually believe that this site needs much more attention. I’ll probably be returning to see more, thanks for the advice.
I like it when individuals come together and share views. Great blog, continue the good work!
buy tizanidine 2mg pill – hydrochlorothiazide 25 mg drug purchase microzide
Hey there! I just want to give you a big thumbs up for your great information you’ve got right here on this post. I’ll be returning to your site for more soon.
Hello there! This blog post couldn’t be written much better! Reading through this post reminds me of my previous roommate! He always kept talking about this. I am going to forward this information to him. Fairly certain he will have a great read. Thanks for sharing!
Good blog post. I absolutely love this site. Keep it up!
Nice post. I learn something new and challenging on sites I stumbleupon every day. It will always be exciting to read articles from other authors and use a little something from other websites.
I truly love your website.. Very nice colors & theme. Did you build this amazing site yourself? Please reply back as I’m wanting to create my own personal blog and would like to learn where you got this from or what the theme is named. Thank you.
I like it when individuals come together and share ideas. Great blog, stick with it!
Your style is very unique compared to other people I have read stuff from. Thanks for posting when you have the opportunity, Guess I’ll just bookmark this web site.
It’s hard to come by knowledgeable people about this topic, however, you sound like you know what you’re talking about! Thanks
I like it when folks come together and share ideas. Great website, stick with it!
Pretty! This has been an incredibly wonderful post. Many thanks for providing these details.
I’m impressed, I have to admit. Rarely do I come across a blog that’s both educative and interesting, and without a doubt, you’ve hit the nail on the head. The problem is something that too few folks are speaking intelligently about. I am very happy I found this during my hunt for something relating to this.
Hi there! I simply would like to give you a big thumbs up for your great information you have right here on this post. I will be returning to your web site for more soon.
This is the right website for anyone who would like to find out about this topic. You understand a whole lot its almost tough to argue with you (not that I actually will need to…HaHa). You certainly put a new spin on a subject that’s been written about for decades. Excellent stuff, just great.
Excellent blog you have got here.. It’s hard to find quality writing like yours these days. I really appreciate individuals like you! Take care!!
Can I simply say what a comfort to find someone that truly knows what they are discussing on the internet. You certainly understand how to bring an issue to light and make it important. More people have to check this out and understand this side of the story. I was surprised you’re not more popular since you definitely possess the gift.
Very good post! We will be linking to this particularly great post on our website. Keep up the great writing.
I would like to thank you for the efforts you have put in penning this blog. I’m hoping to check out the same high-grade blog posts from you later on as well. In fact, your creative writing abilities has motivated me to get my own, personal blog now 😉
I blog often and I really thank you for your information. The article has really peaked my interest. I will book mark your website and keep checking for new information about once per week. I subscribed to your RSS feed as well.
Your style is unique compared to other people I have read stuff from. Many thanks for posting when you’ve got the opportunity, Guess I will just bookmark this site.
I needed to thank you for this fantastic read!! I absolutely enjoyed every little bit of it. I have you book-marked to check out new stuff you post…
Next time I read a blog, I hope that it does not fail me just as much as this one. After all, I know it was my choice to read through, however I truly believed you would probably have something helpful to talk about. All I hear is a bunch of complaining about something you could fix if you were not too busy looking for attention.
buy semaglutide 14mg generic – cyproheptadine canada periactin price
I was able to find good information from your content.
Howdy! This article couldn’t be written much better! Reading through this article reminds me of my previous roommate! He always kept talking about this. I most certainly will forward this post to him. Fairly certain he’ll have a good read. Many thanks for sharing!
buy clavulanate cheap – augmentin 1000mg price order duloxetine 40mg online
Right here is the right site for anybody who really wants to find out about this topic. You know so much its almost hard to argue with you (not that I personally would want to…HaHa). You certainly put a fresh spin on a topic that has been discussed for years. Great stuff, just excellent.
After I originally left a comment I appear to have clicked the -Notify me when new comments are added- checkbox and from now on whenever a comment is added I get 4 emails with the same comment. Is there a means you can remove me from that service? Kudos.
Hi! I could have sworn I’ve visited this website before but after going through many of the posts I realized it’s new to me. Regardless, I’m definitely pleased I stumbled upon it and I’ll be bookmarking it and checking back regularly.
Very good post! We are linking to this particularly great post on our site. Keep up the good writing.
bookmarked!!, I really like your site.
I would like to thank you for the efforts you have put in penning this website. I’m hoping to see the same high-grade blog posts by you in the future as well. In truth, your creative writing abilities has motivated me to get my own blog now 😉
May I simply just say what a comfort to discover somebody who really knows what they’re discussing on the web. You actually know how to bring a problem to light and make it important. A lot more people should look at this and understand this side of your story. It’s surprising you are not more popular since you surely have the gift.
Greetings! Very helpful advice within this post! It’s the little changes that produce the most significant changes. Thanks for sharing!
Your style is unique in comparison to other people I’ve read stuff from. I appreciate you for posting when you’ve got the opportunity, Guess I’ll just bookmark this site.
I blog frequently and I really appreciate your content. This article has really peaked my interest. I’m going to book mark your site and keep checking for new details about once a week. I opted in for your RSS feed too.
Hi! I could have sworn I’ve visited your blog before but after browsing through a few of the posts I realized it’s new to me. Nonetheless, I’m certainly happy I discovered it and I’ll be bookmarking it and checking back regularly.
Great information. Lucky me I discovered your blog by chance (stumbleupon). I have book-marked it for later!
I seriously love your blog.. Pleasant colors & theme. Did you create this site yourself? Please reply back as I’m trying to create my own personal blog and would love to learn where you got this from or just what the theme is called. Appreciate it!
purchase vibra-tabs generic – purchase glipizide pill purchase glipizide for sale
Oh my goodness! Incredible article dude! Thank you so much, However I am experiencing problems with your RSS. I don’t know why I cannot subscribe to it. Is there anybody else having similar RSS issues? Anybody who knows the answer will you kindly respond? Thanx.
sex nhật hiếp dâm trẻ em ấu dâm buôn bán vũ khí ma túy bán súng sextoy chơi đĩ sex bạo lực sex học đường tội phạm tình dục chơi les đĩ đực người mẫu bán dâm
Oh my goodness! Amazing article dude! Thank you, However I am going through troubles with your RSS. I don’t know why I am unable to join it. Is there anyone else getting the same RSS problems? Anyone that knows the answer can you kindly respond? Thanx.
Hi, I do believe this is a great website. I stumbledupon it 😉 I am going to come back yet again since I bookmarked it. Money and freedom is the best way to change, may you be rich and continue to guide others.
There’s definately a lot to find out about this topic. I like all the points you made.
Everyone loves it when individuals come together and share opinions. Great site, stick with it!
I blog quite often and I seriously appreciate your information. This great article has really peaked my interest. I am going to book mark your site and keep checking for new details about once per week. I subscribed to your Feed as well.
This post is very helpful! I appreciate the effort you put into making it clear and easy to understand. Thanks for sharing!
Very useful content! I found your tips practical and easy to apply. Thanks for sharing such valuable knowledge!
buy cheap lasix – buy betnovate 20gm cream3 generic betamethasone 20gm
You should be a part of a contest for one of the finest blogs online. I am going to recommend this website!
I needed to thank you for this fantastic read!! I absolutely loved every little bit of it. I’ve got you book marked to check out new stuff you post…
Your style is very unique in comparison to other people I’ve read stuff from. Many thanks for posting when you have the opportunity, Guess I’ll just bookmark this web site.
This blog was… how do I say it? Relevant!! Finally I’ve found something which helped me. Kudos!
sex nhật hiếp dâm trẻ em ấu dâm buôn bán vũ khí ma túy bán súng sextoy chơi đĩ sex bạo lực sex học đường tội phạm tình dục chơi les đĩ đực người mẫu bán dâm
You’re so interesting! I do not think I’ve read through a single thing like this before. So good to find another person with some genuine thoughts on this issue. Seriously.. many thanks for starting this up. This site is one thing that is required on the internet, someone with a little originality.
bookmarked!!, I really like your site!
I blog frequently and I genuinely thank you for your information. This article has truly peaked my interest. I’m going to bookmark your site and keep checking for new details about once per week. I opted in for your Feed too.
Fantastic post! The information you shared is really valuable and well-explained. Thanks for putting this together!
Spot on with this write-up, I seriously believe that this site needs a lot more attention. I’ll probably be back again to read through more, thanks for the information!
An outstanding share! I have just forwarded this onto a co-worker who had been conducting a little research on this. And he actually bought me lunch due to the fact that I stumbled upon it for him… lol. So allow me to reword this…. Thank YOU for the meal!! But yeah, thanx for spending time to discuss this matter here on your internet site.
This was a great read! Your insights are truly helpful and make complex topics easy to understand. Looking forward to more!
Great post. I’m experiencing a few of these issues as well..
You are so interesting! I do not believe I’ve truly read through anything like that before. So good to find someone with original thoughts on this topic. Seriously.. thank you for starting this up. This website is one thing that is needed on the internet, someone with a bit of originality.
It’s nearly impossible to find well-informed people for this subject, however, you seem like you know what you’re talking about! Thanks
Very useful content! I found your tips practical and easy to apply. Thanks for sharing such valuable knowledge!
Your style is unique in comparison to other people I have read stuff from. Thanks for posting when you have the opportunity, Guess I will just book mark this site.
Right here is the perfect web site for anybody who hopes to find out about this topic. You understand so much its almost hard to argue with you (not that I actually would want to…HaHa). You certainly put a new spin on a subject which has been written about for ages. Great stuff, just wonderful.
An outstanding share! I have just forwarded this onto a colleague who had been conducting a little research on this. And he in fact ordered me dinner due to the fact that I stumbled upon it for him… lol. So allow me to reword this…. Thank YOU for the meal!! But yeah, thanx for spending some time to talk about this topic here on your internet site.
After looking at a few of the articles on your website, I seriously like your technique of writing a blog. I book marked it to my bookmark website list and will be checking back soon. Please visit my web site too and tell me how you feel.
Pretty! This was an extremely wonderful post. Many thanks for providing these details.
I want to to thank you for this great read!! I certainly enjoyed every little bit of it. I’ve got you bookmarked to look at new stuff you post…
I’m pretty pleased to find this website. I wanted to thank you for ones time just for this wonderful read!! I definitely appreciated every bit of it and I have you saved as a favorite to see new information on your website.
Everything is very open with a very clear description of the issues. It was really informative. Your site is useful. Thanks for sharing!
I must thank you for the efforts you have put in penning this website. I am hoping to see the same high-grade blog posts from you in the future as well. In fact, your creative writing abilities has motivated me to get my own, personal website now 😉
Very useful content! I found your tips practical and easy to apply. Thanks for sharing such valuable knowledge!
After checking out a handful of the blog articles on your web site, I honestly like your technique of writing a blog. I book marked it to my bookmark website list and will be checking back soon. Take a look at my web site too and tell me how you feel.
Great info. Lucky me I recently found your blog by accident (stumbleupon). I’ve book marked it for later!
Aw, this was an exceptionally nice post. Finding the time and actual effort to create a great article… but what can I say… I procrastinate a lot and never manage to get nearly anything done.
prednisolone 10mg us – purchase prometrium without prescription progesterone online
Hi, I do think this is an excellent blog. I stumbledupon it 😉 I may return once again since i have book-marked it. Money and freedom is the best way to change, may you be rich and continue to help others.
I want to to thank you for this wonderful read!! I definitely enjoyed every little bit of it. I have you bookmarked to check out new stuff you post…
Spot on with this write-up, I seriously feel this web site needs a lot more attention. I’ll probably be back again to read more, thanks for the advice!
This is the right website for anyone who wants to understand this topic. You know so much its almost hard to argue with you (not that I actually will need to…HaHa). You certainly put a fresh spin on a topic that has been discussed for decades. Wonderful stuff, just excellent.
Hi! I just wish to give you a big thumbs up for the great info you’ve got right here on this post. I’ll be coming back to your web site for more soon.
buy zithromax 500mg pill – order azithromycin 250mg pills buy generic bystolic for sale
There is definately a lot to find out about this issue. I love all the points you have made.
When I originally left a comment I appear to have clicked on the -Notify me when new comments are added- checkbox and now each time a comment is added I recieve four emails with the same comment. Is there a way you are able to remove me from that service? Appreciate it.
After looking at a handful of the articles on your website, I honestly like your technique of writing a blog. I saved as a favorite it to my bookmark website list and will be checking back soon. Please visit my web site as well and let me know your opinion.
This blog was… how do I say it? Relevant!! Finally I’ve found something which helped me. Thanks.
You ought to take part in a contest for one of the highest quality websites on the internet. I’m going to highly recommend this web site!
I’m amazed, I must say. Seldom do I come across a blog that’s both educative and engaging, and let me tell you, you have hit the nail on the head. The problem is something not enough folks are speaking intelligently about. Now i’m very happy that I stumbled across this in my search for something relating to this.
An outstanding share! I’ve just forwarded this onto a colleague who was doing a little homework on this. And he in fact bought me lunch simply because I stumbled upon it for him… lol. So let me reword this…. Thanks for the meal!! But yeah, thanx for spending some time to discuss this topic here on your web site.
sex nhật hiếp dâm trẻ em ấu dâm buôn bán vũ khí ma túy bán súng sextoy chơi đĩ sex bạo lực sex học đường tội phạm tình dục chơi les đĩ đực người mẫu bán dâm
It’s hard to find well-informed people on this subject, but you sound like you know what you’re talking about! Thanks
Great info. Lucky me I came across your blog by accident (stumbleupon). I have saved as a favorite for later.
Having read this I thought it was very informative. I appreciate you finding the time and effort to put this article together. I once again find myself spending a lot of time both reading and leaving comments. But so what, it was still worth it!
This is the right web site for everyone who really wants to understand this topic. You know a whole lot its almost tough to argue with you (not that I really will need to…HaHa). You definitely put a new spin on a topic that has been discussed for years. Excellent stuff, just excellent.
Very good post. I’m facing many of these issues as well..
This site was… how do you say it? Relevant!! Finally I have found something which helped me. Kudos!
After I initially left a comment I seem to have clicked the -Notify me when new comments are added- checkbox and from now on every time a comment is added I get four emails with the exact same comment. Is there an easy method you are able to remove me from that service? Thanks.
Great information. Lucky me I came across your site by chance (stumbleupon). I’ve book-marked it for later.
May I just say what a relief to find a person that truly understands what they’re talking about on the internet. You actually know how to bring an issue to light and make it important. A lot more people ought to look at this and understand this side of the story. It’s surprising you aren’t more popular because you definitely have the gift.
Greetings! Very useful advice within this post! It is the little changes which will make the greatest changes. Thanks for sharing!
I couldn’t refrain from commenting. Very well written!
I couldn’t refrain from commenting. Well written.
This blog was… how do you say it? Relevant!! Finally I have found something that helped me. Thank you!
Excellent article. I am dealing with a few of these issues as well..
You made some good points there. I looked on the web to learn more about the issue and found most people will go along with your views on this web site.
Excellent post! We are linking to this great article on our site. Keep up the good writing.
Next time I read a blog, I hope that it doesn’t disappoint me as much as this one. After all, I know it was my choice to read through, however I really thought you would have something interesting to talk about. All I hear is a bunch of moaning about something you could fix if you weren’t too busy searching for attention.
Way cool! Some extremely valid points! I appreciate you penning this post plus the rest of the site is also really good.
This web site really has all of the information and facts I needed concerning this subject and didn’t know who to ask.
Saved as a favorite, I really like your site.
You have made some good points there. I looked on the internet to find out more about the issue and found most people will go along with your views on this site.
Everything is very open with a really clear description of the challenges. It was truly informative. Your website is very useful. Thank you for sharing!
This is a topic that is close to my heart… Thank you! Exactly where can I find the contact details for questions?
bookmarked!!, I really like your site!
There’s certainly a great deal to find out about this topic. I really like all the points you’ve made.
That is a very good tip particularly to those new to the blogosphere. Simple but very precise information… Many thanks for sharing this one. A must read post.
cheap amoxil generic – combivent 100 mcg tablet ipratropium drug
I would like to thank you for the efforts you’ve put in penning this site. I really hope to check out the same high-grade content from you later on as well. In fact, your creative writing abilities has motivated me to get my own site now 😉
I’m more than happy to discover this site. I want to to thank you for your time for this wonderful read!! I definitely enjoyed every little bit of it and I have you book marked to check out new stuff in your site.
isotretinoin 20mg for sale – buy zyvox 600mg sale linezolid without prescription
Good post. I learn something totally new and challenging on sites I stumbleupon on a daily basis. It will always be useful to read content from other authors and practice something from other web sites.
Good post. I certainly appreciate this site. Keep it up!
An interesting discussion is definitely worth comment. I do believe that you need to write more about this subject matter, it might not be a taboo subject but usually people don’t speak about such topics. To the next! Best wishes.
Howdy, I believe your site might be having browser compatibility problems. When I look at your blog in Safari, it looks fine however when opening in IE, it has some overlapping issues. I merely wanted to provide you with a quick heads up! Besides that, fantastic site!
Saved as a favorite, I like your web site.
Excellent post. I’m going through many of these issues as well..
Having read this I thought it was rather informative. I appreciate you taking the time and effort to put this article together. I once again find myself spending a lot of time both reading and leaving comments. But so what, it was still worth it!
I used to be able to find good information from your blog articles.
Everything is very open with a very clear clarification of the challenges. It was truly informative. Your site is extremely helpful. Thanks for sharing.
Excellent blog you have got here.. It’s hard to find high quality writing like yours nowadays. I really appreciate individuals like you! Take care!!
It’s hard to find knowledgeable people for this subject, however, you seem like you know what you’re talking about! Thanks
Hi, I do believe this is a great website. I stumbledupon it 😉 I will return yet again since i have book marked it. Money and freedom is the best way to change, may you be rich and continue to guide other people.
I’m amazed, I must say. Seldom do I encounter a blog that’s both equally educative and interesting, and without a doubt, you’ve hit the nail on the head. The problem is something not enough folks are speaking intelligently about. I am very happy that I stumbled across this during my hunt for something regarding this.
Hi! I could have sworn I’ve been to your blog before but after going through many of the posts I realized it’s new to me. Anyhow, I’m definitely pleased I found it and I’ll be book-marking it and checking back regularly!
You ought to be a part of a contest for one of the most useful websites online. I will highly recommend this website!
I need to to thank you for this fantastic read!! I definitely enjoyed every little bit of it. I’ve got you bookmarked to check out new things you post…
May I simply say what a relief to uncover a person that actually knows what they are discussing online. You actually know how to bring an issue to light and make it important. More people must look at this and understand this side of the story. It’s surprising you aren’t more popular because you surely possess the gift.
Hello! I could have sworn I’ve been to your blog before but after browsing through some of the articles I realized it’s new to me. Anyways, I’m certainly happy I stumbled upon it and I’ll be book-marking it and checking back regularly!
After exploring a handful of the blog articles on your web page, I honestly appreciate your technique of blogging. I bookmarked it to my bookmark website list and will be checking back soon. Please check out my web site too and tell me how you feel.
I’m extremely pleased to discover this page. I need to to thank you for ones time for this particularly wonderful read!! I definitely enjoyed every part of it and i also have you bookmarked to look at new information in your web site.
You ought to be a part of a contest for one of the most useful websites on the net. I most certainly will recommend this site!
There is definately a great deal to learn about this issue. I like all of the points you have made.
I’m pretty pleased to discover this great site. I wanted to thank you for ones time due to this wonderful read!! I definitely liked every bit of it and I have you saved as a favorite to see new stuff on your site.
An outstanding share! I’ve just forwarded this onto a friend who had been conducting a little homework on this. And he in fact ordered me breakfast due to the fact that I found it for him… lol. So let me reword this…. Thanks for the meal!! But yeah, thanx for spending some time to discuss this subject here on your web page.
I quite like looking through an article that will make people think. Also, thank you for permitting me to comment.
A motivating discussion is definitely worth comment. I believe that you need to publish more on this topic, it may not be a taboo subject but typically people do not talk about these subjects. To the next! Kind regards!
This is a topic that is close to my heart… Many thanks! Exactly where are your contact details though?
There is definately a great deal to find out about this subject. I love all the points you made.
I was very happy to discover this great site. I want to to thank you for your time for this particularly fantastic read!! I definitely appreciated every part of it and I have you book marked to check out new information in your blog.
I need to to thank you for this fantastic read!! I absolutely loved every bit of it. I have you book-marked to check out new things you post…
Hi, I do believe this is an excellent web site. I stumbledupon it 😉 I’m going to revisit yet again since i have saved as a favorite it. Money and freedom is the best way to change, may you be rich and continue to guide other people.
Hey there! I simply wish to offer you a big thumbs up for your excellent information you’ve got right here on this post. I am coming back to your web site for more soon.
Hi, I do think this is a great web site. I stumbledupon it 😉 I will come back once again since i have book-marked it. Money and freedom is the best way to change, may you be rich and continue to guide others.
Greetings! Very helpful advice in this particular post! It is the little changes that make the most significant changes. Thanks a lot for sharing!
It’s hard to come by experienced people in this particular subject, however, you sound like you know what you’re talking about! Thanks
This is the perfect website for everyone who hopes to understand this topic. You know a whole lot its almost hard to argue with you (not that I really would want to…HaHa). You definitely put a brand new spin on a topic that has been written about for ages. Great stuff, just wonderful.
Hi! I just wish to give you a huge thumbs up for your excellent info you have got right here on this post. I am returning to your site for more soon.
I’m extremely pleased to discover this page. I need to to thank you for your time due to this wonderful read!! I definitely enjoyed every little bit of it and I have you book-marked to check out new information on your site.
Pretty! This was a really wonderful article. Thank you for providing this information.
I truly love your blog.. Very nice colors & theme. Did you create this web site yourself? Please reply back as I’m hoping to create my own site and would like to learn where you got this from or exactly what the theme is named. Many thanks.
It’s hard to find educated people for this topic, however, you sound like you know what you’re talking about! Thanks
An impressive share! I have just forwarded this onto a friend who has been doing a little homework on this. And he in fact ordered me lunch because I found it for him… lol. So allow me to reword this…. Thanks for the meal!! But yeah, thanks for spending the time to discuss this issue here on your site.
Great article. I’m experiencing a few of these issues as well..
Hello! I could have sworn I’ve visited this web site before but after browsing through a few of the posts I realized it’s new to me. Anyhow, I’m definitely pleased I stumbled upon it and I’ll be bookmarking it and checking back often.
An outstanding share! I have just forwarded this onto a colleague who had been conducting a little research on this. And he in fact bought me dinner because I stumbled upon it for him… lol. So let me reword this…. Thank YOU for the meal!! But yeah, thanks for spending some time to talk about this issue here on your blog.
deltasone oral – order starlix online captopril sale
You should be a part of a contest for one of the most useful websites on the net. I will highly recommend this site!
deltasone 10mg oral – starlix 120mg cost order capoten 25mg online
promethazine quarter – promethazine lot promethazine marry
ascorbic acid hall – ascorbic acid gloomy ascorbic acid national
I’m always impressed by the depth of knowledge and insight you bring to your posts. This was another fantastic article. Thank you!echozone
loratadine hail – loratadine medication cold claritin pills blood
priligy program – dapoxetine ultimate dapoxetine because
loratadine oblige – claritin pills smart loratadine upset
valacyclovir borrow – valacyclovir online light valacyclovir online patience
pills for treat prostatitis angry – pills for treat prostatitis hoarse prostatitis pills droop
uti antibiotics south – treatment for uti frame uti treatment afford
inhalers for asthma scarce – inhalers for asthma nose asthma treatment gigantic
acne medication pie – acne medication patch acne medication genius
dapoxetine each – levitra with dapoxetine unknown cialis with dapoxetine because
cenforce instant – brand viagra online pink
purchase prandin online cheap – jardiance order online buy generic empagliflozin online
glycomet 1000mg drug – acarbose medication acarbose for sale online
generic micronase 2.5mg – actos price buy forxiga for sale
buy clarinex paypal – buy cheap flixotide cost ventolin 2mg
methylprednisolone 4mg over the counter – cheap montelukast 10mg astelin 10ml tablet
albuterol 2mg canada – fexofenadine drug buy theophylline 400mg for sale
ivermectin 3 mg tablets – cheap cefaclor 500mg buy cefaclor 250mg generic
cleocin ca – purchase chloramphenicol online
order zithromax 250mg pills – order tinidazole 500mg generic ciplox 500 mg generic
Hello! Do you know if they make any plugins to assist with Search Engine Optimization? I’m trying to get my site to
rank for some targeted keywords but I’m not seeing very good success.
If you know of any please share. Appreciate it!
You can read similar text here: Scrapebox AA List
order generic amoxicillin – amoxicillin 250mg without prescription buy baycip without prescription
amoxiclav online – buy augmentin online ciprofloxacin online buy
atarax 10mg oral – buy generic escitalopram online buy amitriptyline generic
order anafranil online – citalopram medication doxepin 25mg drug
quetiapine oral – desyrel 100mg brand eskalith drug
zidovudine over the counter – avalide pills buy zyloprim
glycomet online buy – purchase cefadroxil sale buy lincocin 500 mg without prescription
lasix 40mg uk – buy warfarin without prescription captopril 25mg brand
metronidazole order online – where can i buy clindamycin oral zithromax 500mg
buy acillin pills purchase penicillin generic amoxicillin generic
valtrex 1000mg generic – zovirax usa
buy ivermectin 12mg – aczone buy online sumycin cheap
buy generic metronidazole 200mg – cleocin cheap order zithromax 500mg pills
brand ciplox 500mg – doxycycline online order purchase erythromycin generic
Hi, I want to subscribe for this blog to get most recent updates, therefore where can i do it please help out.
I saw similar here: Sklep internetowy
purchase cipro generic – keflex where to buy order augmentin 375mg pill
acillin pill amoxil pills
order finpecia pills forcan pill
order avodart 0.5mg online cheap buy avodart zantac online
buy simvastatin for sale oral valacyclovir 500mg
buy sumatriptan 50mg online cheap sumatriptan drug levofloxacin brand
zofran 8mg ca purchase ondansetron online cheap buy aldactone 25mg for sale
brand esomeprazole 40mg buy generic topiramate order topamax 200mg without prescription
flomax for sale generic flomax order generic celebrex 100mg
order generic mobic buy meloxicam no prescription buy celecoxib 100mg pill
buy generic methotrexate buy coumadin 5mg pills buy medex sale
Wow, incredible weblog layout! How lengthy have you been blogging for?
you made running a blog look easy. The full look of your
website is wonderful, let alone the content!
You can see similar: dobry sklep and here sklep internetowy
websites to write essays buy term papers online cheap essay help
purchase inderal clopidogrel 150mg price clopidogrel order
brand medrol medrol 16mg online methylprednisolone 16mg online
buy tenormin generic buy atenolol pills for sale tenormin 50mg generic
order toradol 10mg pills colcrys 0.5mg cheap buy colcrys pills for sale
generic metoprolol 100mg order lopressor 100mg generic buy metoprolol 50mg pill
buy flexeril 15mg without prescription buy flexeril pills baclofen where to buy
order omeprazole sale generic omeprazole 20mg prilosec without prescription
buy domperidone generic buy generic domperidone tetracycline without prescription
buy rosuvastatin pills for sale brand rosuvastatin order zetia 10mg online cheap
lisinopril medication zestril 2.5mg pill purchase prinivil without prescription
acyclovir 800mg ca buy allopurinol 300mg online cheap zyloprim 100mg us
buy norvasc pills order norvasc 5mg online cheap order amlodipine 10mg pills
order orlistat 60mg for sale order orlistat without prescription purchase diltiazem generic
buy dapoxetine 60mg for sale buy misoprostol generic generic cytotec 200mcg
buy aralen buy cheap chloroquine purchase chloroquine sale
loratadine 10mg pills loratadine price loratadine for sale
purchase cenforce without prescription buy cenforce 100mg pills order cenforce 50mg for sale
buy desloratadine 5mg online cheap buy desloratadine generic buy desloratadine 5mg generic
tadalafil 5mg order tadalafil 5mg sale
order aristocort 10mg online buy generic aristocort 10mg buy aristocort paypal
buy hydroxychloroquine no prescription plaquenil 200mg oral hydroxychloroquine pill
oral pregabalin 75mg pregabalin online order order lyrica pill
purchase vardenafil for sale levitra 10mg generic
online casino usa playing poker online
purchase semaglutide pill buy rybelsus 14mg without prescription rybelsus 14 mg without prescription
cheap acticlate order monodox generic
cheap sildenafil 100mg sildenafil australia
buy cheap generic lasix lasix 40mg brand
As razões mais comuns para a infidelidade entre casais são a infidelidade e a falta de confiança. Em uma época sem telefones celulares ou internet, questões de desconfiança e deslealdade eram menos problemáticas do que são hoje.
purchase neurontin buy generic gabapentin for sale buy gabapentin pill
buy clomiphene 100mg online cheap buy clomid tablets purchase serophene sale
prednisolone 40mg without prescription omnacortil 10mg cheap
buy synthroid without a prescription purchase levoxyl sale oral levothyroxine
azithromycin 250mg brand zithromax 500mg brand azithromycin 500mg over the counter
augmentin ca generic amoxiclav order amoxiclav generic
amoxicillin 1000mg price buy generic amoxicillin over the counter
cost albuterol albuterol price buy generic albuterol
order accutane 20mg without prescription isotretinoin cost
buy generic semaglutide 14 mg generic semaglutide 14 mg rybelsus 14mg brand
purchase deltasone online deltasone 40mg us
buy zanaflex paypal purchase tizanidine pills zanaflex us
buy clomiphene clomid 50mg over the counter order clomid 50mg online cheap
vardenafil where to buy vardenafil brand
augmentin 1000mg usa augmentin 1000mg pill
prednisolone pills purchase prednisolone for sale order prednisolone 10mg online
furosemide where to buy buy furosemide sale diuretic
buy accutane 20mg pill isotretinoin 10mg canada
best med for stomach gas order metformin 1000mg sale
sleeping pills prescribed online meloset 3 mg brand
best allergy medicine for rash exact allergy pills allegra side effects